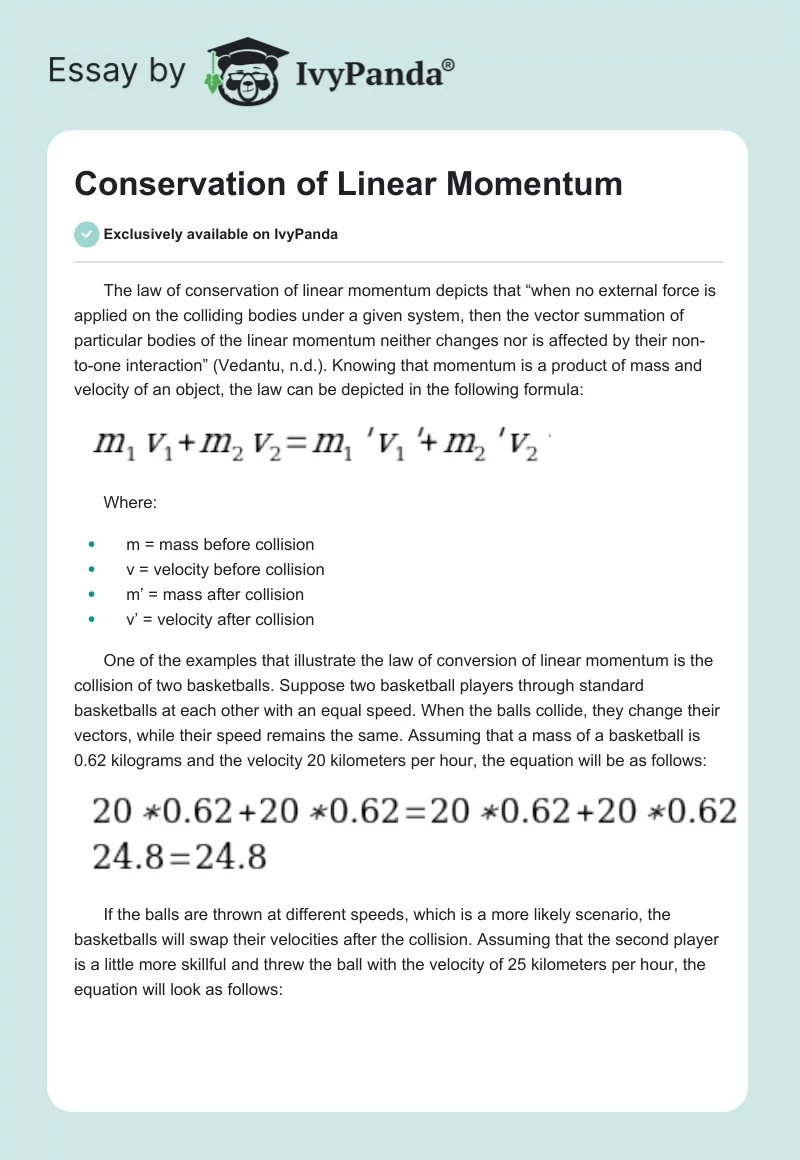
The law of conservation of linear momentum depicts that “when no external force is applied on the colliding bodies under a given system, then the vector summation of particular bodies of the linear momentum neither changes nor is affected by their non-to-one interaction” (Vedantu, n.d.). Knowing that momentum is a product of mass and velocity of an object, the law can be depicted in the following formula:
Where:
- m = mass before collision
- v = velocity before collision
- m’ = mass after collision
- v’ = velocity after collision
One of the examples that illustrate the law of conversion of linear momentum is the collision of two basketballs. Suppose two basketball players through standard basketballs at each other with an equal speed. When the balls collide, they change their vectors, while their speed remains the same. Assuming that a mass of a basketball is 0.62 kilograms and the velocity 20 kilometers per hour, the equation will be as follows:
If the balls are thrown at different speeds, which is a more likely scenario, the basketballs will swap their velocities after the collision. Assuming that the second player is a little more skillful and threw the ball with the velocity of 25 kilometers per hour, the equation will look as follows:
The equation demonstrates that even though the velocities of the balls changed after the collision, the linear moment remained unchanged for the system.
It is crucial to notice the described system does not exactly fit the model, as there are several forces that act upon the basketballs. For instance, the balls are affected by gravity and the force of friction. However, the collision still qualifies for demonstrating the law conservation of linear momentum, as the influence of these external forces is minimal.
Reference
Vedantu. (n.d.). Conservation of linear momentum. Web.