Abstract
Statistical reasoning is built on a foundation of probability, thus an understanding of statistical basics is linked to probability concepts and vice versa. This essay presents an array of quantitative data (The number of phone calls you get in each morning and in each afternoon) and a brief account of descriptive statistics. Finally how to interpret the statistical results of the data provided.
A brief account on descriptive statistics
Descriptive statistics have three functions; besides being a mathematical expression of a population’s data characteristics, it measures the central tendency of data. Central tendency is how the data collected aggregate around a most typical value. For this purpose, three values are essential; first, the mean, which is the average value of a data column. The second is the median, which is the middle observation of a data column. Finally is the mode, which is the most frequent value in a data column. The mean value is the most affected by changes in sample size (Krzanowski 2007).
The third function of descriptive statistics is to measure data dispersion, which means data spread or variability, around the mean value. For this purpose, three measures are important; 1- the range, which is the difference between the highest and lowest values in a data column. Second, the standard deviation, which quantifies the inconsistency of data around the mean value; third, the variance, which expresses the observed differences from what is expected if the data are not normally distributed. It is a square value (s 2) and equals ∑(X – X~)2 / (n-1) where X is the observed value and X~ is the mean value and (n) is the sample size (Dekking et al 2005).
Standard error of the mean measures how the sample means coincides with the population mean, it is needed to calculate the confidence intervals for the population mean. It equals standard deviation / the square root of (n) where n is the sample number). Whereas standard deviation measures the variability in a single sample, the standard error of the mean estimates the variability between two or more samples (Krzanowski 2007).
Skewness is the extent of data asymmetry; it helps in the initial evaluation of data normality. Positive skewness means a tendency of data to be near the low side of values. Negative skewness means the opposite, while non-skewed data means data fit a normal distribution. The word kurtosis means curvature and it is a measure pointing to the extent of observed values’ distribution peak. It provides information about how high the data peak is compared to the value of its standard deviation. The most common reason for measuring kurtosis is to determine whether or not data are consequential to a normally distributed population. Kurtosis is often described within the framework of the following three general categories, all of which are depicted by representative frequency distributions (Sheskin 2004).
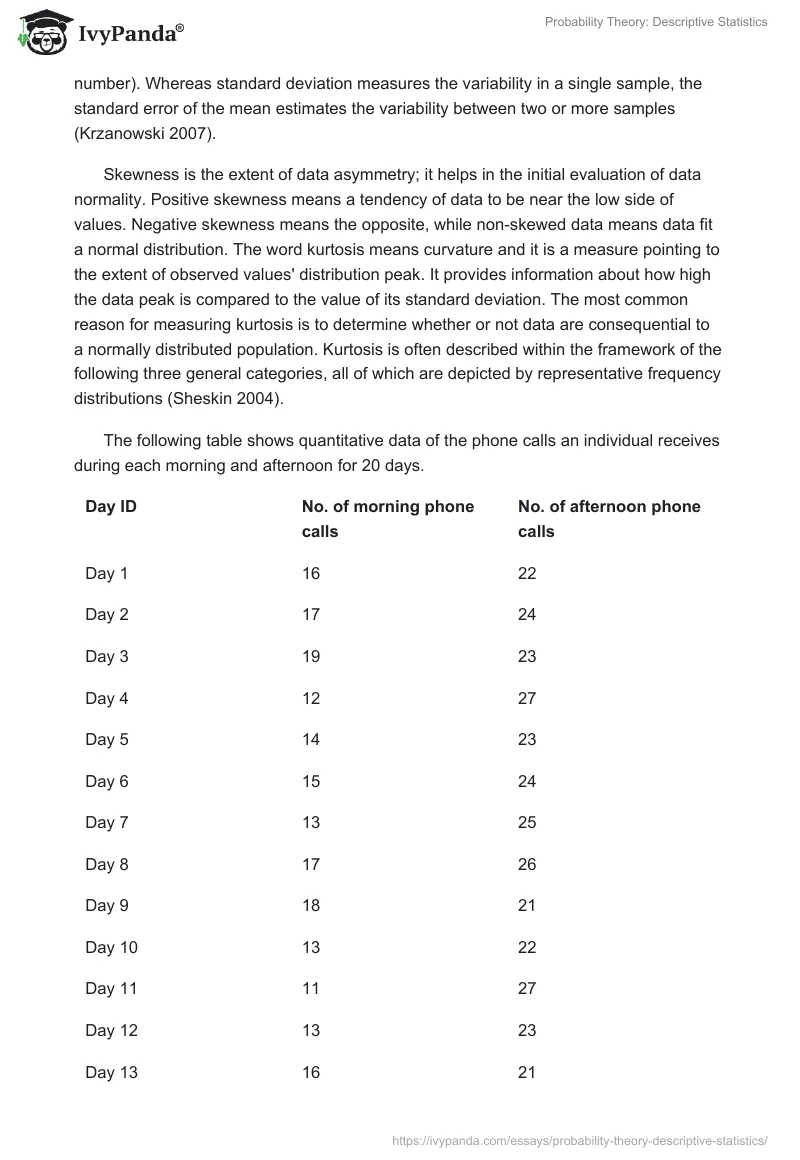
The following table shows quantitative data of the phone calls an individual receives during each morning and afternoon for 20 days.
Descriptive statistics
The next table show the descriptive statistics of the data provided.
Table legend: Var = Variable, Mo_C = Morning calls, Af_C= Afternoon calls. SE_M= standard error of mean, St, Dev= standard deviation, Skw=Skewness, and Kurto= Kurtosis.
Interpretation
In both columns, the median value is less than the mean, so data do not typically fit a normal distribution. Skewness shows that in the morning calls data, there is a shit to the left (tendency towards low values) and in the morning calls data, there is a shift to the right (tendency towards high values). Kurtosis of morning calls data points to the possibility of having a higher peak than if normally distributed, while kurtosis of afternoon calls points to the data distribution is flatter than if normally distributed. Variance is an expression of how data differ from the mean in abnormal data distribution (Pipkin 1986).
References
Dekking, F. M., Kraaikamo, C., Lopuhaa, H. P., and Meester, L. E (2005). A Modern Introduction to Probability and Statistics: Understanding Why and How. Delft, Netherlands: Springer.
Krzanowski, W. J (2007). Statistical Principles and Techniques in Scientific and Social Investigations. New York: Oxford University Press.
Pipkin, F. B (1986). Medical Statistics Made Easy. Philadelphia: W. B. Saunders.
Sheskin, D. J (2004). Handbook of Parametric and Nonparametric Statistical Procedures (3rd edition). Boca Raton, Fl: Chapman & Hall/CRC.