CAPM Model
The required rate of return will be computed using the capital asset pricing model (CAPM). The model is an identity that calculates the required rate of rate by adding a risk premium to the risk free rate of return. The model takes into account risks arising from the market in which the asset trades.
The systematic risk is represented by the beta factor. It gives the amount of risk. The risk premium is the product of price of risk and the amount of risk (Atrill, 2009). The formula for the capital asset pricing model is illustrated by the equation shown below.
Cost of equity = risk free rate of return + beta * risk premium
Risk free rate
Risk free rate is the rate of interest that does not have risks such as interest risk fluctuations, default risk, re-investment risk, and currency fluctuations. For the analysis, the interest rate for a 10 year US treasury bond will be the risk free rate of return (Bloomberg L.P., 2013).
Beta
The beta of an asset measures the volatility of a company’s stock relative to the changes in the market. Computation of asset beta is founded on historical returns and thus may not give an estimate of the firm’s future share prices due to the dynamism of the prevailing market conditions (Vance, 2003).
Risk premium
Risk premium can be defined as the incentives for investing in a risky asset. The risk premium is the amount over and above the risk free rate of return.
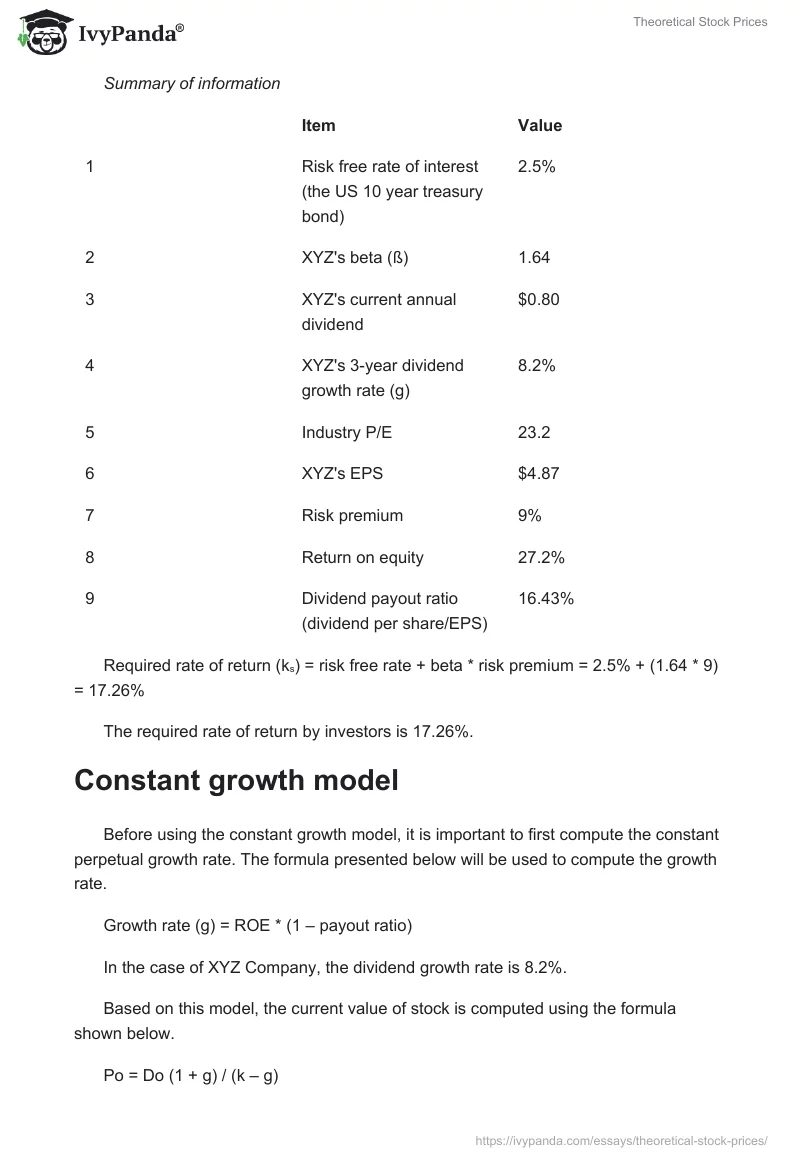
Summary of information
Required rate of return (ks) = risk free rate + beta * risk premium = 2.5% + (1.64 * 9) = 17.26%
The required rate of return by investors is 17.26%.
Constant Perpetual Growth Rate
Before using the constant growth model, it is important to first compute the constant perpetual growth rate. The formula presented below will be used to compute the growth rate.
Growth rate (g) = ROE * (1 – payout ratio)
In the case of XYZ Company, the dividend growth rate is 8.2%.
Based on this model, the current value of stock is computed using the formula shown below.
Po = Do (1 + g) / (k – g)
- The recent annual dividends paid by the company (Do) amounted to $0.80
- Constant perpetual growth rate (g) = 8.2%
- Required rate of return k = 17.26%
Po = 0.80 (1 + 0.082) / (0.1726 – 0.082) = 0.8656 / 0.0906 = $9.554
The theoretical value of the current stock of the company is $9.554. It can be observed that the stock of XYZ Company is trading at a price higher than the intrinsic value. Thus, the shares of the company are overvalued.
Causes of the Differences Between the Current Stock Quote and Theoretical Prices
There is a difference between the current stock quote (P = $76.28) of XYZ stock and the theoretical price (Po = $9.554). The differences are caused by a number of factors. The first factor is the differences in time period. The theoretical price is based on historical information of the company while the current price is often based on current information about the company.
The second factor is the differences in the composition of the market portfolio used in the calculations. The third factor is the difference in the approaches used to calculate the value of beta (Kapil, 2010). Thus, the difference in the valuation methods also contributes to the differences in the two prices. The fourth factor is that buying and selling of stock in the stock market affect the current stock prices (Collier, 2009).
Thus, the current stock price is subjected to constant changes in various variables unlike the theoretical price. Finally, the prevailing market conditions make the current stock prices to change towards a certain given trend while the theoretical price remains fairly stable over a long period of time. This creates the difference in the two prices.
Price/Earning (P/E) Model
Based on the model,
Stock price per share = P/E ratio * Earnings per share
15.65 * 4.87 = $76.2155
Thus, based on the P/E model, the price per share is $76.2155. The price obtained using this method is not similar to the price obtained using CGM because P/E model is based on current market conditions while CGM is based on historical and future market conditions.
New prices at a risk premium of 12%
Required rate of return (ks) = risk free rate + beta * risk premium = 2.5% + (1.64 * 12%) = 22.18%
The required rate of return will increase from 17.26% to 22.18%.
Constant Growth Model
Po = Do (1 + g) / (k – g)
Po = 0.80 (1 + 0.082) / (0.2218 – 0.082)
= 0.8656 / 0.1398 = $6.19
With an increase in risk premium, the theoretical value decreases to $6.19.
P/E model
Stock price per share = P/E ratio * Earnings per share
15.65 * 4.87 = $76.2155
The prices of the shares will no change when using the P/E model.
References
Atrill, P. (2009). Financial management for decision makers. Harlow, England: Prentice Hall.
Bloomberg L.P. (2013). World markets: Bonds. Web.
Collier, P. (2009). Accounting for managers. London: John Wiley & Sons Ltd.
Kapil, S. (2010). Financial management. India: Person Education.
Vance, D. (2003). Financial analysis and decision making: Tools and techniques to solve. United States: McGraw-Hill books.