Introduction
Gravity-powered machines, or trebuchets, have long been considered a famous engineering invention of the Middle Ages, used to throw weapons of war. These weapons could be stones, other heavy objects, or flammable liquids. Trebuchets were helpful in a war until the invention of gunpowder (Karlsson 2). The trebuchet should be seen as a more advanced catapult device because the physics of this mechanism allows for launching projectiles at a farther distance. The advancements include the development of the hinged counterweight, which allowed the addition of powder and reduced the machine loading time between shots (Karlsson 1). For this reason, trebuchets began to be used, including not only in the military.
However, the 20th and 21st centuries have had several technological developments that have made gravity-powered machines of little use, even in the military. Their range of use today ends at entertainment and academic purposes. In physics and mathematics, they are used to study motion, and in history, they are used to study past human inventions, technological changes, and achievements. The popularity of trebuchets is mainly due to their simplicity: the energy of the sharply falling weight, attached to the short end of the lever, is transferred to the loose core, which due to the resulting momentum, is transported over long distances. Of interest in this context is the relationship between the projectile weight and the horizontal distance traveled by the projectile. The present work investigates how the weight affects the throw range and whether there are any limitations in this connection.
Trebuchet Principles
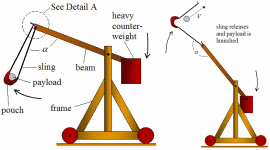
The common type of trebuchet is the counterweight trebuchet that operates on the principles of gravity and leverage. There is a main axle of the trebuchet, and from it, a long lever (throwing arm) is suspended. A framework of support is referred to as bents that hold the main axle in place, and the bent allows it to rotate in blocks on both sides. The setting of the throwing arm is such that the longer side is about three times or more than the length of the shorter side (Bertrand 3). The counterweight is attached on the shorter side of the throwing arm, and in some cases, the weight is suspended; hence the name hinged counterweight trebuchet.
The machine is started by pulling down the long side of the throwing arm, and the hinged counterweight on the shorter side is raised. There is a pouch on which the projectile is loaded. The pouch has two cords, and one of them is fixated on the tip of the long arm. The projectile is loaded and placed in a trough under the trebuchet. The second cord is then attached to a pin on the long arm. Notably, the loose attachment allows the cord to slip during the throw (Bertrand 3). When the machine is released, the counterweight falls through the displaced height, and this causes rotation of the throwing arm. Consequently, the pouch is whirled around, and the second cord slips, causing the pouch to open, releasing the projectile.
The experiment’s hypothesis states that an increase in the projectile’s weight causes a decrease in the distance traveled by the projectile. The law of energy conservation, also known as the first law of thermodynamics, posits that energy cannot be created, destroyed, or altered but can be transformed from one form to another (Haddad 1). In simpler terms, it means that energy is always conserved. In the trebuchet setting, the counterweight is suspended from the ground at a specific distance. Therefore, through the suspension, the counterweight gains gravitational potential energy. If it is assumed that at ground level, the counterweight has zero potential energy, then at a height displacement of h, the gravitational potential energy will be:
Eg = m1Δh
Eg is the gravitational potential energy, m1 is the counterweight mass, g is the gravitational pull, and Δh is the displaced height. On starting the trebuchet, the counterweight moves through distance h, and the gravitational potential energy is converted to kinetic energy (Ek). Therefore:
Eg = Ek
It is this kinetic energy that is used to propel the projectile. If an assumption is made that all potential gravitational energy is converted to kinetic energy, then:
Where m2 is the projectile mass, and v is the projectile’s velocity. Therefore, as the mass of the projectile increases, the equivalent velocity at which it travels in the air is reduced, and consequently, it will travel a shorter distance. Moreover, the increase in weight necessitates more energy to lift the projectile against gravitational pull before it is fired. We can thus say that the potential gravitational force of the counterweight mass is equal to the potential gravitational force and the projectile’s kinetic energy. This can be presented in an equation as shown:
The above formulas demonstrate that the gravitational potential energy remains constant irrespective of the projectile mass. Since the sum of energy on either side of the equation has to be equal, an increase in the projectile mass will cause a decrease in the projectile’s velocity, and thus the mass travels a shorter distance.
The interest in the study of this paper originates primarily from a personal interest in learning more about the mechanics of this medieval invention. It is intriguing how engineers of the Middle Ages could use technology at a primitive level without digital processing and machine data like today. They also had the exquisite hands-on skill to develop such a machine. In addition, personal motivation sparks from a desire to test one’s hypotheses dictated by logical thinking. Notably, it is expected that the greater the projectile weight, the shorter the throwing range, but there must be some limitations in this algorithm, which is also of interest to this study.
Research Question
What effect does the weight of the projectile from the trebuchet mechanism have on the projectile range? Additionally, are there any constraints that directly or indirectly affect this relationship?
Variables
The present experimental work investigates the relationship between the projectile weight and the horizontal distance from the trebuchet, and therefore it is possible to assume which variables are used in the study. The independent variable that is manipulated in this work is the weight of the projectile from the trebuchet. This is a quantitative quantity measured in kilograms: a change in the weight value is expected to affect the range of the projectile. In this case, the dependent variable is the projectile range. In the absence of other factors, the projectile release weight is expected to affect the dependent variable directly. Finally, the control variables that are not subject to change throughout the experiment are the projectile material, the tribute material, the “weather conditions,” the measurement scales, and the projectile angle. In addition, all other characteristics of the trebuchet, except the projectile range.
Risk Assessment
It should be understood that the trebuchet experiment was performed in an online format, whereby a simulation was used as the actual experiment. Therefore, the experiment did not pose any risk or ethical issues.
Image of a real trebuchet

Method
For this work, the VirtualTrebuchet 2.0 Gravity Throwing Machine Simulator is used to adjust the setup parameters and observe the results manually. This simulator was used to manipulate the launch angle and investigate the effect of these manipulations on the projectile’s range. The characteristics of the trebuchet of interest in the project are rigidly fixed and recorded to ensure the accuracy and consistency of the recorded data in case of accidental web page updates. The metric system chosen is meters and kg as SI Units (SI Units). Figure 4 below shows the initial parameters that are set for the first run of the Trebuchet: The highlighted fragments are the characteristics manipulated throughout the experiment.

Input Data
The present experiment measures quantitative data that show the dependence of one variable on another. The best to visualize such a dependence — the change in throw distance as the release angle changes — is a table. Table 2 below shows the results obtained by measuring the throw distance of various masses of the projectiles with a diameter of 1 meter at a 45-degree angle of release. Note that three measurements for each angle are given to minimize data errors. It is also worth noting that the weights used ranged from 20 kgs to 140 kgs.
Table 2. Results of the virtual experiment.
Data Processing
Table 2 presents the raw data collected from the experiment. The data is in pairs of values, which means that a suitable model for their processing would be mapping the points on a Cartesian coordinate system. First of all, it should be said that it is impossible to achieve complete unbiased data since all three measurements for each projectile weight resulted in identical results. Apparently, VirtualTrebuchet 2.0 is programmed with equations whose values depend precisely on the input parameters, making it impossible to achieve data diversity. For this reason, averaging and finding mean errors is not possible for this set of numbers. In addition, either a regular scatter plot or a regression model can be used to predict the results if they have the property of linearity.
Presentation of the Processed Data
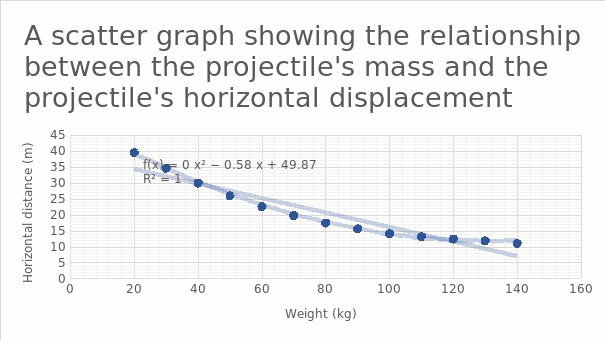
Using MS Excel, a scatter plot of the dependence of the throw range on the initial projectile weight launched was constructed. The projectile weight values and horizontal distances were placed on the x and y axes, respectively. The visualization of this relationship is shown in Figure 5. As it can be seen, the graph for the data is not an actual straight line but is a curve that slopes from the left to the right side
Analysis of the Graph
From the graph, it is clear that the weight of the projectile influences the distance it travels. The gradient has a negative value, which suggests that an increase in the projectile weight (independent variable) causes a decrease in its horizontal distance (dependent variable). Thus, the horizontal distance traveled by the projectile has an inverse relationship with the projectile’s weight. This agrees with the project’s hypothesis, which states that increasing the projectile’s weight causes a decrease in the distance traveled by the projectile. A line of best was included in the graph, and it clearly denotes that the two variables do not have any direct proportionality, and consequently, there is no linear relationship.
The decrease in the horizontal distance was rapid between 20 and 100 kgs. As from 100 kgs forward, the slope of the curve reduces, and the decrease in the distance becomes more gradual. This hints that after 100 kg, any additional weight on the projectile decreases the distance traveled by a smaller amount. The graph has a coefficient of determination R2 value of 0.9983. This figure is critically high and denotes the accuracy of the experiment. It is evident that if the plotted curve is extended on both ends, it will not cross either axis. The equation of the trendline (y = 0.0022x2 + 0.58x + 49.868) indicates that the maximum horizontal displacement is 49.9. However, this is not true because the graph lacks a linear trend.
Conclusion
In summary, several important conclusions were found about the trends found in the data processing of this experiment. First, the throwing range of the projectile has an inverse dependence on the weight of the projectile. At smaller weights, the effect of the masses on the decrease of horizontal distance is rapid, while at large weights, the influence is little. Therefore, smaller projectile masses should be used to attain longer horizontal displacements. Second, several other factors should be kept constant to achieve maximum displacement. They include the angle of displacement, the lengths of both the short and long arms, lengths of the weight and sling, the pivot’s height, the projectile’s material, and most importantly, the counterweight mass.
Evaluation and Improvement
The interaction with the experiment design on the simulation link was seamless. The values were easier to manipulate, and the results were accurate. However, in future iterations of this or similar work, it is recommended to use a more accurate, systematic scale for measurement. Instead of randomly selecting a given weight, off-the-shelf software can be used for more accurate measurement: it can be an interval with steps as small as 1 gram. This sensitivity is supposed to give more reliable results and reduce the error. Moreover, the system should simulate a more practical experiment such that it does not offer the same results when several trials are done using a specific value for a given variable. Availability of different results on the same variable will help to reduce experimental errors and thus increase precision.
In addition, for future works, it is suggested to complicate the study design and measure the effect of several independent variables on the final result. In addition to the projectile’s weight, this could be the length of the short arm of the lever or the angle of displacement as additional characteristics affecting the variable. Measurements of these variables will aid in determining the optimum values that will achieve the maximum horizontal distance of the projectile.
If another opportunity arises to perform the experiment, it will be better suited to carry out a practical experiment. In the simulator, some factors such as the speed of the wind, friction force (due to air resistance) as the projectile travels, and other environmental conditions are not accounted for; thus, the results do not represent actual figures in a natural setting. This will also allow for the investigation of other variables such as wheels. Wheels were one of the modifications made to the medieval trebuchets. They were used to support the mechanism when the counterweight was released and prevent it from shaking the base. When in motion, the wheels increase the trebuchet’s power, which is likely to increase the velocity of the projectile. Moreover, the wheels allowed the trebuchet to be fired while in motion. Therefore, this creates an interesting topic to further research and understand the relationship between the wheels, the trebuchet’s motion, and the distance traveled by the projectile. This could be impossible to achieve when using the online trebuchet simulation.
Works Cited
Bertrand, Daniel A. “Building the Medieval Trebuchet.” 2019, pp. 1 – 59, Web.
Haddad, Wassim M. “Introduction.”A Dynamical Systems Theory of Thermodynamics, SCH-School edition, Princeton University Press, 2019, pp. 1–38, Web.
Karlsson, Daniel. “The Trebuchet: Longer Shots with Wheels?”. 2018, pp. 1– 21, FYGB08 Web.
“Trebuchet Physics.”Real World Physics Problems, Web.
“SI Units.” NIST, 28 Sep. 2021, Web.


