Problem
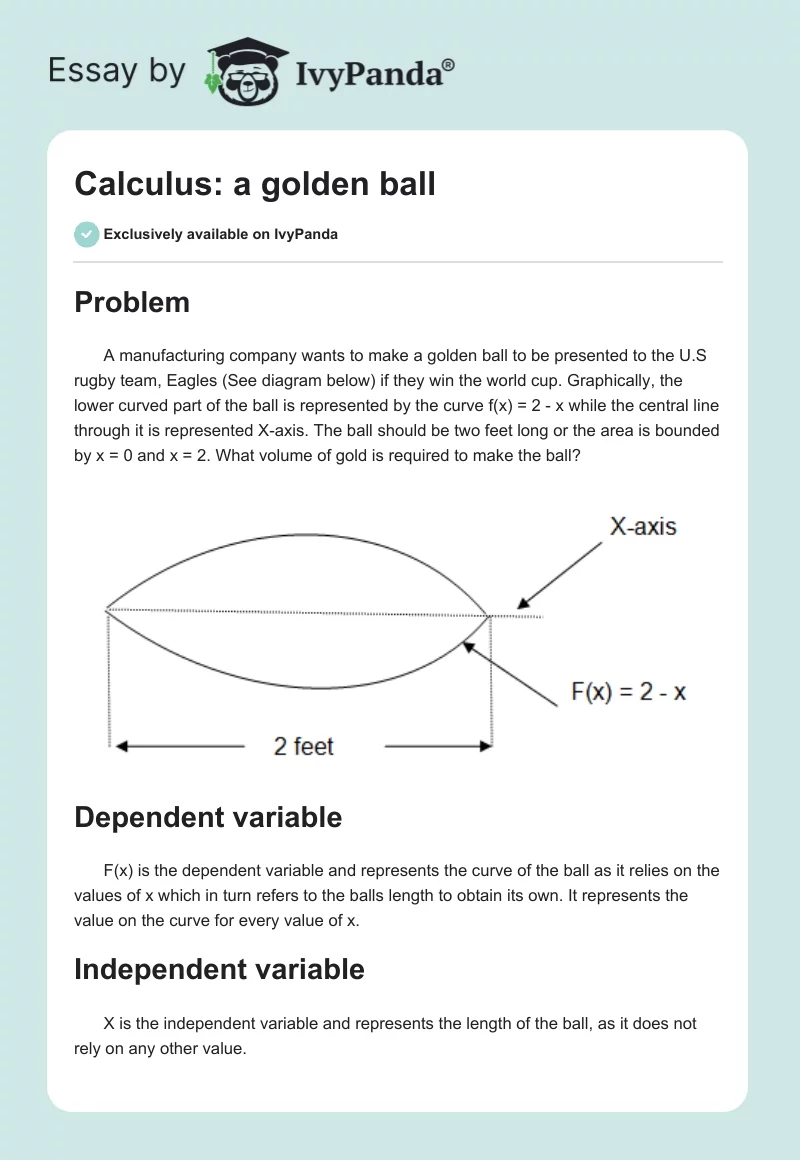
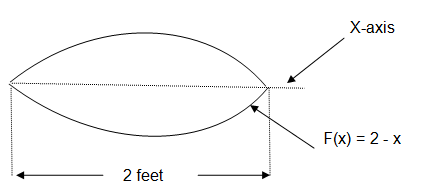
A manufacturing company wants to make a golden ball to be presented to the U.S rugby team, Eagles (See diagram below) if they win the world cup. Graphically, the lower curved part of the ball is represented by the curve f(x) = 2 – x while the central line through it is represented X-axis. The ball should be two feet long or the area is bounded by x = 0 and x = 2. What volume of gold is required to make the ball?
Dependent variable
F(x) is the dependent variable and represents the curve of the ball as it relies on the values of x which in turn refers to the balls length to obtain its own. It represents the value on the curve for every value of x.
Independent variable
X is the independent variable and represents the length of the ball, as it does not rely on any other value.
Domain
This represents values that the length of the ball can take. It is between zero and two feet. The maximum length required being 2 feet.
Range
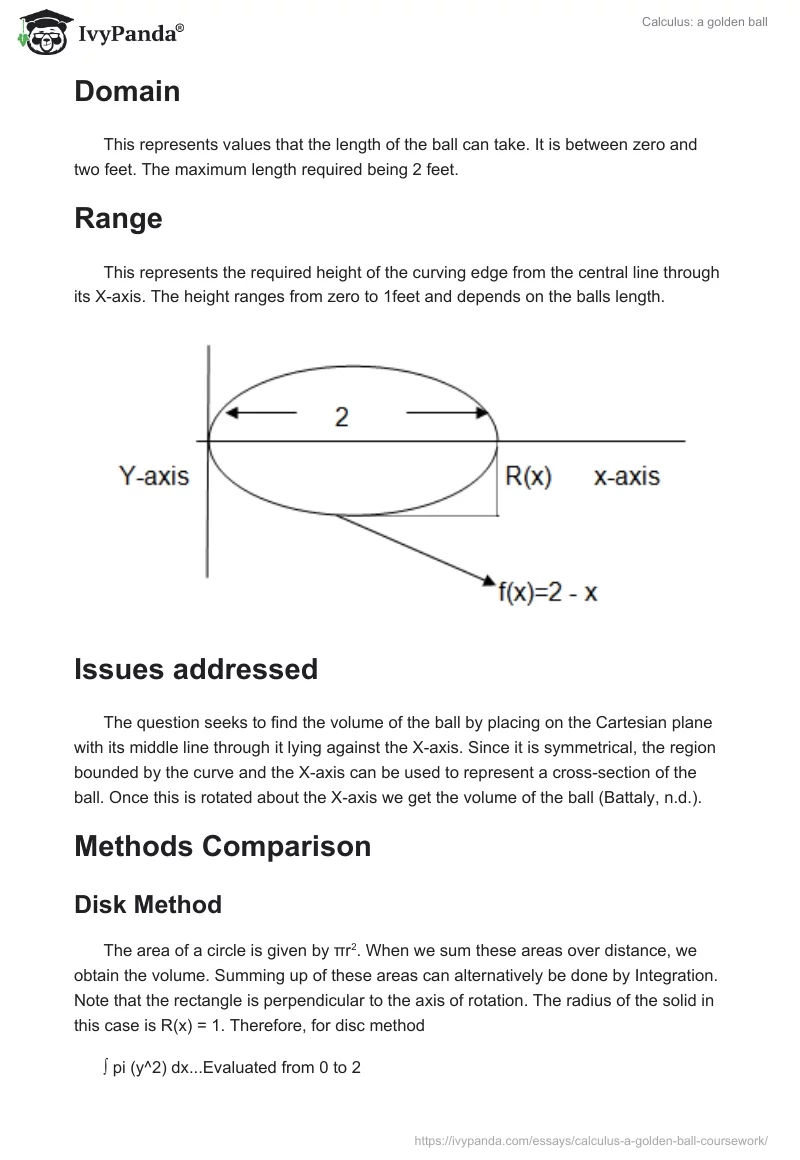
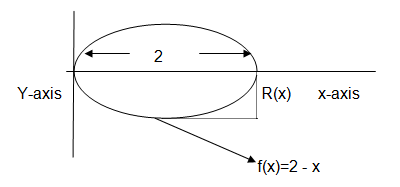
This represents the required height of the curving edge from the central line through its X-axis. The height ranges from zero to 1feet and depends on the balls length.
Issues addressed
The question seeks to find the volume of the ball by placing on the Cartesian plane with its middle line through it lying against the X-axis. Since it is symmetrical, the region bounded by the curve and the X-axis can be used to represent a cross-section of the ball. Once this is rotated about the X-axis we get the volume of the ball (Battaly, n.d.).
Methods Comparison
Disk Method
The area of a circle is given by πr2. When we sum these areas over distance, we obtain the volume. Summing up of these areas can alternatively be done by Integration. Note that the rectangle is perpendicular to the axis of rotation. The radius of the solid in this case is R(x) = 1. Therefore, for disc method
∫ pi (y^2) dx…Evaluated from 0 to 2
∫ pi (2 – x)^2 dx
Result is 8pi/3
Shell method
Our rectangle of reference is vertical to the x-axis thus: For this method the golden ball folds into a crown in “3 d.” The circumference will just be 2*pi*y times height x thickness dy which in these terms would be 2*pi*y*(2 – x) dy. The reason why the assumption ends up with 2 – x is because the created crown is bigger at one end yet thins out on the other side.
r(y) = y
L(y) = 2 – x
The problem can be simplified by shifting to the equivalent problem of y=x rotated about x axis with x from 0 to 2. Eventually, 2 – x actually just reduces to y. So circumference equals 2pi*y^2 dy. Evaluating this from 0 to 2 the result is 8pi/3.
Thus, the methods give the same answer.
Relationship between differentiation and integration
These two processes are the reverse of each other. When we differentiate a function we obtain its slope. On integrating the slope we obtain the initial parent function. The relationship can be used in the above questions to work the initial problems given. This implies that differentiation and integration successfully undo each other. In fact, the derivative of an integral of any given function normally gives the original function.
For example in part A, the integration process can be used to get the equation for distance covered from that of velocity. When S= 10t-7t2+3t3 is differentiated, we get ds/dt=10-14t+9t2. However, if ds/dt=10-14t+9t2 is integrated for instance ∫ ds/dt=10-14t+9t2 we obtain S=10t-14t2/2+9t3/3. Hence, S = 10t-7t2+3t3 which is the original function.
In simple terms therefore, differentiation is the mechanism of finding the rate of change of distance (velocity) while integration of the velocity function gives the distance covered. Repeated differentiation can determine the maximum acceleration which is analogous to local minimum or maximum point in any moving object.
Distance got through integration is analogous to a path of function, volume and surface area of graph functions. In general terms, differentiation might be applicable in finding the rate of change of things while integration might be used to find distance, areas and volumes.
Fundamental theorem of Calculus
A steel manufacturing company is tasked with the production of curved steel bars used in the building of bridges. In order to develop the prototypes, the development team has to develop an equation that fits the requirements of the final bar. The equation identified to define the curve is sin x+ 10. The design outcome is shown in the following graph below. Calculate the area of the curve between π/2 and π?
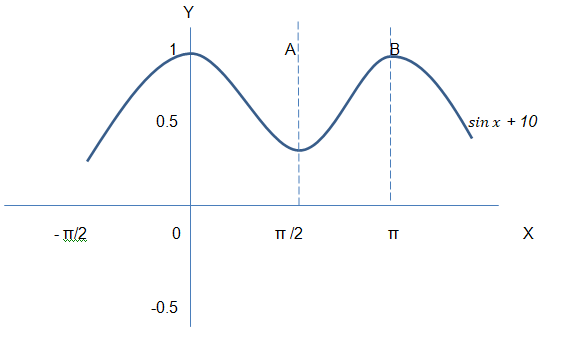
From the problem, the dependent variables are the values on the X-axis while the independent variables are the values on the Y-axis. The domain (values of the independent variable) of the problem is the X-axis interval the curve occupies; Domain (π/2 π).
The range is a set of values of Y-axis specified by all values in the domain and includes zero to maximum value. Based on the parameters of the problem, the range could explicitly assume Y values between 0 and 1.
The specific issue the problem is addressing is about finding the area of the curve under the closed and open intervals. The manufacturing company wants to achieve the specification of the steel bar that would meet the design requirements. The issue is to find the exact values of the prototype relative to the actual bar.
First Fundamental Theorem of Calculus states that; if f is a continuous real-valued function on a closed interval (a, b) and F is a function defined for all values in the interval (a, b), then F is continuous on the interval and differentiable on the open interval and the derivative of F equals f (Stewart, 2009). This first part can be used to find the area of the curve on the closed interval.
Area =
We know that the derivative of the area equal the function of the curve. In simple terms, F’(x) = f(x) =sin x +10
The second Fundamental Theorem of Calculus states that; if f is a real valued function defined on a closed interval (a, b) that divulge an anti-derivative ‘g’ on the same interval and is integrable on the interval, then the integration of f equals the difference of the function g (a) and g (b) (Stewart, 2009).This second part can be used in our problem to find the area of the curve on the open interval.
Area =
=
=
= 1
Answer key
- The function is continuous for all points between π/2 and π. If F’(x) = f (x) then the function of the curve will be the derivative of the area function.
- Area equals the integration of the curve function =
Therefore, between the interval π/2 and π, the area is the difference between two functions
according to 2nd fundamental theorem of calculus.
Comparison and importance of the Methods
The two methods used above differ in that the first method applies only to continuous functions while the second method applies continuous and discontinuous function as long as an anti-derivative g exists. The two parts of fundamental theorem of calculus have been used to find the area of the curve in the problem.
Fundamental Theorem of calculus is thus important in approximating the area from derivatives and anti-derivatives of curve functions. Generally, the fundamental theorem of calculus enables us to work out the relationship between area and length of curved objects in engineering designs. The design specifications of curved objects are relative to mathematical values obtained from the application of the two theorems.
Fibonacci sequence
F (n) = a*
+ b*
but F(0) = a + b = 0 and F(1) = 2.62a – 0.62b = 1
By solving the two simultaneous equations we find a = 0.45 and b = -0.45
Therefore F (n) = 0.45*
– 0.45*
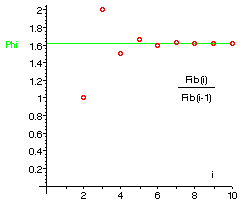
From the graph, it is clear that Phi convergence to a specific number. The number Phi converges to approximately 1.6.
References
Battaly, G. (n.d). Volumes of Revolution Shell Method.New York, NY: Westchester Community College.
Stewart, J. (2009). Calculus: Concepts and Contexts. Florence, KY: Cengage Learning.