Corporate Financial Management: Project Management Report

Workings

Cost of capital (COC) = 10%

Depreciation: straight-line method

The projects are mutually exclusive

To evaluate the project, the company should consider the use of project viability tools as follows:

Net present value

Project 1

Cost = $100,000

Expected income =

• Year 1 = 29000
• Year 2 = (1000)
• Year 3 = 2000

Residue Value = 7,000

Calculating depreciation:

Depreciation= cost – residual value/ Useful life

= (100,000 -7000)/3 = 32,000

When using C.O.C. of 10% then:

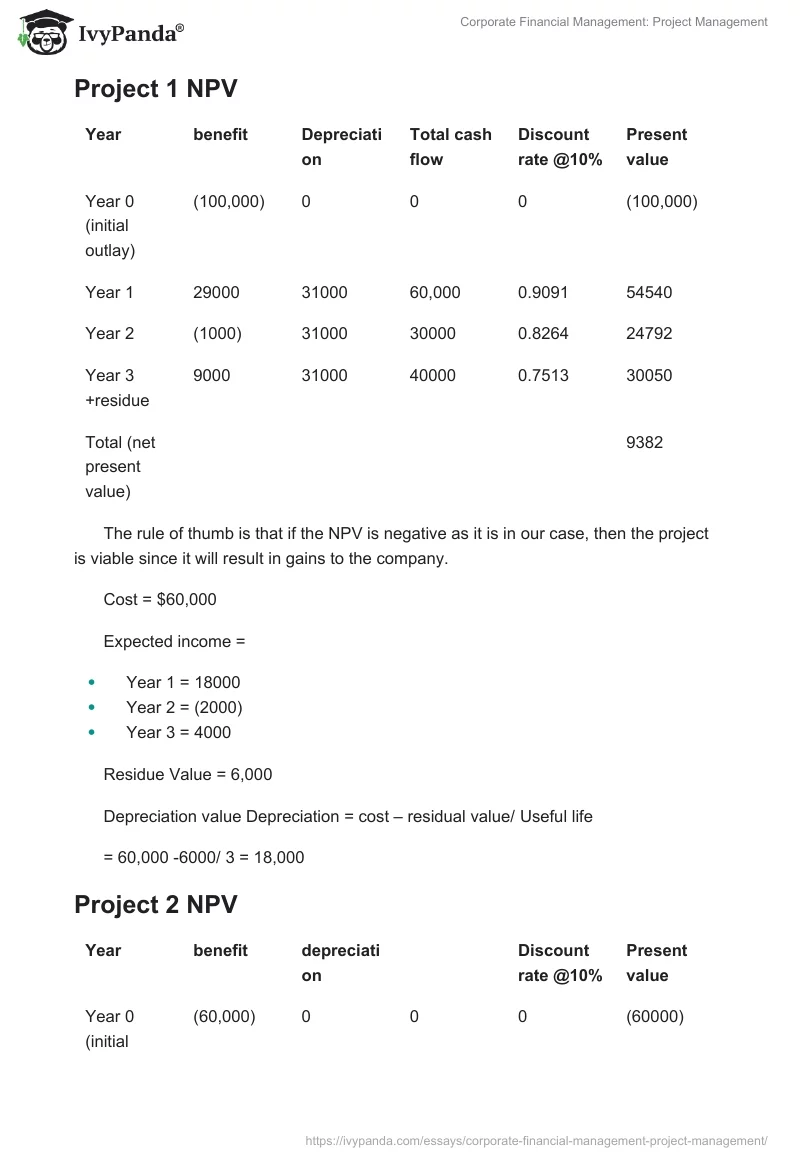
Project 1 NPV

The rule of thumb is that if the NPV is negative as it is in our case, then the project is viable since it will result in gains to the company.

Cost = $60,000

Expected income =

• Year 1 = 18000
• Year 2 = (2000)
• Year 3 = 4000

Residue Value = 6,000

Depreciation value Depreciation = cost – residual value/ Useful life

= 60,000 -6000/ 3 = 18,000

Project 2 NPV

The result of the project NPV is negative; this is to imply that the project is viable thus; the company should not invest in it. It will lead to an overall gain to the company.

NPV Result analysis

From the calculations above, project 1 is more viable than project 2, it gives a higher rate of return at 9382; the project gives a rate of 6985 (Gary, 2010)

Approximate IRR

Project 1

Using the trial and error method

Assume that:

1. the highest present value be w₁
2. rate of return used in 1 above be Y
3. second-highest rate of return be w₂
4. rate of return used in 3 above be x
5. the intermediary figure of y and X be z (IRR)
6. Cost of venture c. = 100,000

then IRR = (Z/W₂-C) = [(Y-X)/(W₂-W₁)] + Y

If NPV AT 10% at 10 % is 9360 choose a higher rate and get the NPV let us use 20%

Let the rate be X 15%

Then

Let Y be 20%

PV@ LDR – I₀ (HDR – LDR)

IRR = LDR + PV @ LDR –PV @ HDR

=10 + 109,360 – 100.000 ( 20 – 10)

109,360 – 93960 = 16.08%

The rate of return (IRR) of the project is higher than the expected rate of return of 10%, thus, the project is viable (Wheelen and Hunger, 1998)

Project 2

1. the highest present value be w₁
2. rate of return used in 1 above be Y
3. second-highest rate of return be w₂
4. rate of return used in 3 above be x
5. the intermediary figure of y and X be z (IRR)
6. Cost of venture c. = 100,000

Then IRR = (Z/W₂-C) = [(Y-X)/(W₂-W₁)] + Y

If NPV AT 10% at 10 % is 6968 choose a higher rate and get the NPV let us use 20%

The rate at 20%

IRR = 10 + (66968 – 60,000)/ (66968 -57304 )*(20 -10)

IRR = 17.21 %

The rate of return (IRR) of the project is higher than the expected rate of return of 10%, thus the project is viable.

Analysis: IRR

Project 2 is better it has a higher IRR

Payback method

The payback method of analyzing projects has been interpolated as the easiest and straightforward method of project analysis. The method is simply looking at the period that a certain project is going to take to recoup the amount of money that the investor has invested (Shane, 2003).

Project 1

40,000 = 1 year 10,000 ÷ 40000 = 0.25

10,000 =?

Thus Payback period is 2+0.25=2.25 Years

Project 2

60,000 will be recovered within 2 years for the first 52,000 and the remaining 8,000 from the third year.

28,000 = 1 year 8,000 ÷ 28,000 = 0.29

8,000 =?

Thus, Payback period of project 2 is 2+0.29=2.29 Years

Analysis: Payback Method

Project 1 is a better investment since it has a lower payback time.

According to the analysis using NPV, IRR, and Payback method, none of the projects is viable; this is so because the financial return of the project is negative. The major role of a company when making a certain investment is to have economic gain from the company; in this case, both the projects are giving negative returns, thus at the end of the process, they will leave the company worse off than it was when they were being implemented. None of the projects should be implemented. For a project to be viable, the NPV value should be higher than or equal to Zero; the IRR should be lower than the cost of capital (expected rate of return), and it should be having a viable Payback period; both our projects lack these parameters (Livingston, 2008)

The method that I find more appropriate to use when calculating the viability of a project is the NPV method; when using net present value, it takes into account the duration that the project is likely to be in operation then discount the income using the cost of capital, if the NPV is a positive number, then a project is viable.

N.P.V. = (discounted outflows – initial capital) (Long and Plosser,1983).

When undertaking a project, the initial outlay plus the running expenses need to be adequately covered by a projected income. Capital outlaw in a business is an important aspect to consider when starting up a project, the capital initially employed in the business should be recouped at the end of the project and still give some benefit to the company: when considering the recouping care should be taken to take into accounts the time value of money. Net present value helps to interpolate the present value of future income. When the N.P.V. means that, the project can cover all the expenses that have been incurred when undertaking it. Expenses to be considered in a venture are both initial capital expenses and running costs; the method is realistic.

The challenge facing the NPV method of evaluation is that the value of money keeps deteriorating; incorporating the deterioration of money when gauging a project is wise and gives a more accurate value and quality information to evaluate a project (Marcus,2010)

Using net present value is a more superior and better method than just calculating the net cash outflow since it takes into account the future value of money and is a straightforward way of evaluating the viability of a project. The method also takes into account the entire period of a project and thus it analyses a project as a whole. The method is faced with some problems since it assumes that only the cost of capital affects the value of money, this is an assumption since various factors affect the value of money. The method also assumes risks. The business environment is full of risks and thus it is not logical for the method to assume that the business will operate in a risk-free environment (Wynant, 1980)

When the NPV method is used to compare more than one project, it ranks projects according to their profitability; a rational businessperson will undertake the project that has high returns. When projects are ranked according to their profitability, it gives management an easy task in making an investment decision.

The following are the advantages of the NPV method:

• it incorporates the future value of money when evaluating a project
• it is a straightforward way of evaluation
• it has a realistic approach
• it offers reliable information when comparing more than one project (Pons, 2008).

References

Gary, L. 2010. project management theory and practice.Baca Raton: Auerbach publishers.

Livingston, J., 2008. Founders at work: stories of startups’ early days. Berkeley: Apress.

Long, J. and Plosser,C.I., 1983. Real Business Cycles. Chicago: University of Chicago Press.

Marcus, G. 2010. Fundamental of agile project management: an overview. New York: ASME Press.

Pons, D., 2008. Project management for new product development. Project Management Journal, 39(2), pp. 82-97.

Shane, S., 2003. A General Theory of Entrepreneurship: the Individual-Opportunity. Northampton: Edward Elgar Publishing.

Wheelen, L. and Hunger, J.,1998. Strategic Management and Business Policy: Entering 21st Century Global Society. Massachusetts: Addison Wesley.

Wynant, L., 1980. Essential elements of project financing. Harvard Business Review, 58(3), pp.165-173.