Introduction
It is necessary to carry out financial analysis on capital investments. A project manager should compare various attributes of different projects before deciding which project to pursue. This is because capital investments require a large amount of initial capital outlay. Secondly, the benefits from such investments flow in for a long period.
Thirdly, capital investment decisions in most cases deal with an organization’s optimal capital structure in terms of debt and equity (Brigham & Ehrhardt 2010). This is because an organization has to find a source for funds to finance the investment. Therefore, these projects are irreversible. Finally, capital is a limited resource. Most companies or nations cannot afford to invest in all projects. Resources should be dedicated to ventures with high returns. Therefore, care should be taken when appraising and ranking projects.
Capital budgeting is a multi-faceted process with distinctive stages. A commonly used model is comprised of strategic planning, making accept or reject decisions, project implementation and monitoring, and project implementation audit (Dayananda, Irons, Harrison, Herbohn, & Rowland 2002).
The essence of this procedure is to ensure that a company gets maximum returns from the project within a reasonable time. When carrying out capital budgeting, it is necessary to take into account the time value of money. This is because $1 may not have the same value in five years to come. This is because of the fluctuations in the economy, such as inflation and exchange rate fluctuations, among others.
Therefore, when evaluating projects, a project manager needs to express expected future stream cash flow in terms of the present value. Some of the capital investment projects include construction of new building, purchase of a building and a new machinery, among others. This work compares the viability of two projects using various tools with an attempt to select the most viable project.
Comparison of project A and project B
A project manager should assess the viability of a venture before injecting capital. Several approaches can be used to evaluate the feasibility of a project. A common approach is the net present value. Other approaches include payback period, return on investment, profitability index, internal rate of return, and accounting rate of return, among others. These approaches use expected streams of cash flows (Brigham & Ehrhardt 2010). Explanation of some of the tools is given below.
Net present value
Brigham and Ehrhardt (2010, p. 383) define net present value as the “present value of a project’s cash inflow minus the present value of its costs” (Brigham & Ehrhardt 2010). This approach shows how much a project contributes to the shareholders’ funds. High returns will result in an increase in share prices.
An advantage of the net present value is that it is easy to compute. A major drawback of this approach is that it is sensitive to changes in the discount rate. A slight change in the discount rate may affect accept or reject decision to be made. Secondly, net present value depends on future streams of income. The future performance of a venture is uncertain.
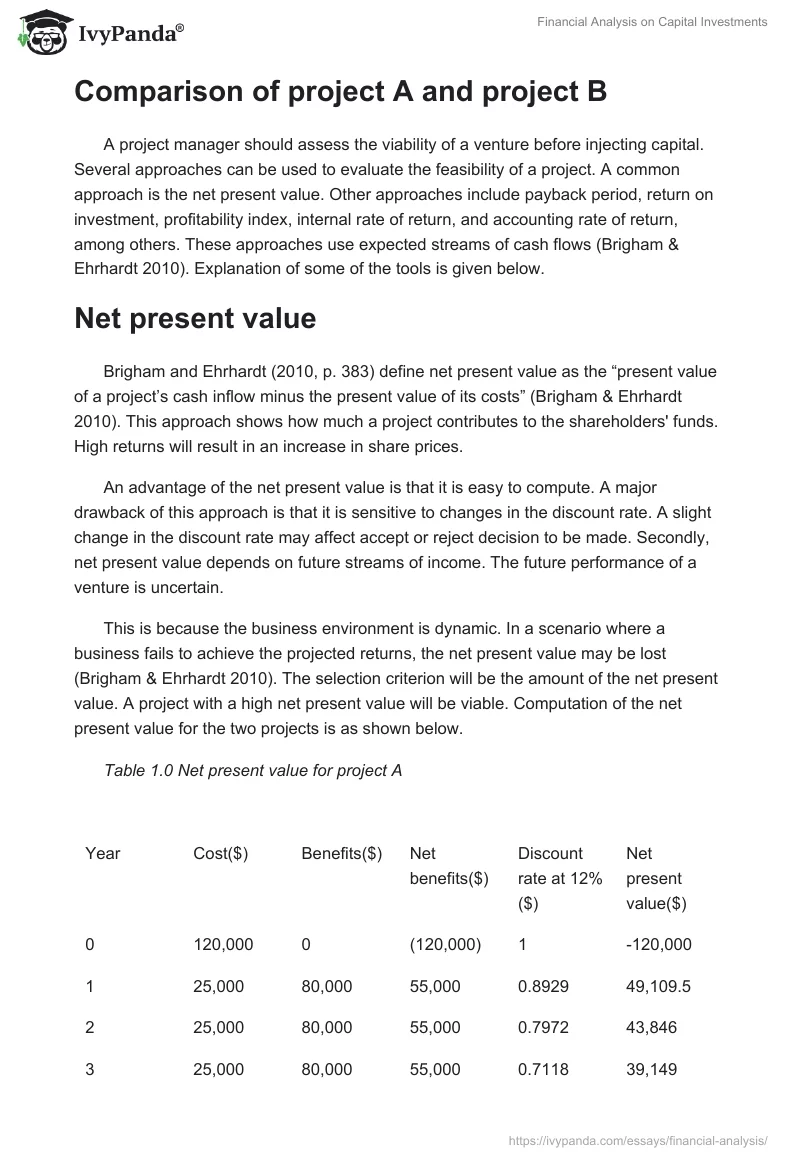
This is because the business environment is dynamic. In a scenario where a business fails to achieve the projected returns, the net present value may be lost (Brigham & Ehrhardt 2010). The selection criterion will be the amount of the net present value. A project with a high net present value will be viable. Computation of the net present value for the two projects is as shown below.
Table 1.0 Net present value for project A
Table 1.1 Net present values for project B
From the above tables, the net present value for project A is $12,104.5, while for project B, it is $(31, 962.00). From the computations, we note that project A has a positive net present value, while project B has a negative project value. Therefore, project A gives higher returns than project B. The decision criteria will be to accept projects with a positive net present value. A project manager will select project A for investment because it gives positive returns.
Payback period
Brigham and Ehrhardt (2010, p. 411) define payback period as “the number of years required to recover a project cost” (Brigham & Ehrhardt 2010). They further state that this approach has a number of shortcomings. “It ignores cash flows beyond the payback period, it does not consider the time value of money and it does not have a precise acceptance rule” (Brigham & Ehrhardt 2010).
This approach does not give an indication of a project’s risk and liquidity. A project manager may use discounted payback period so as to take care of time value of money. The decision criterion is to accept a project with a shorter payback period. This approach is useful because it defines the duration in which a project ties capital. Payback period is obtained through a division of the initial investment cost by the annual cash flows (Brigham & Ehrhardt 2010).
Project A
Initial investment outlay – $120,000
Annual cash flow – $55,000
Therefore, the payback period is 120,000/55,000 = 2.18 years/
Project B
Initial investment outlay – $80,000
Annual cash flow – $20,000
Therefore, the payback period is 80,000/20,000 = 4 years.
From the calculations above, project A has a payback period of 2.18 years. The payback period for project B is 4 years. Since the decision criterion is to select a project with shorter payback period, an investor will choose to invest in project A because it has a shorter payback period.
Return on investment
Owners of a company invest capital so as to get returns. They expect such returns to be increasing over time. Besides, all phases of operations should generate this profitability. On the other hand, creditors are interested in the ability of the company to make timely loan repayment and interest.
Management of a company should manage the shareholders investments and produce profits. Shareholders and creditors use return on investment to assess the ability of a company to generate adequate return (Brigham & Ehrhardt 2010). This approach can also be used to compare the returns from various projects.
This approach does not match returns with the costs. Such information aids management in decision making. The decision rule for return on investment is to select a project with a higher rate of return (Brigham & Ehrhardt 2010). Computation of return on investment is as shown below.
Return on investment = Gains – Investment costs
Investment costs
Return on investment for project A:
Gains – $165,000
Investment cost – $120,000
Gains – Investment costs $45,000
Therefore, return on investment is 45,000/120,000 = 37.5%.
Return on investment for project B:
Gains – $60,000
Investment cost – $80,000
Gains – Investment costs $ (20,000)
Therefore, return on investment is (20,000)/80,000 = -25.0%.
From the above calculations, the return on investment for project A is 37.5% while for project B, it is -25.0%. Project B has negative return on investment. Therefore, a project manager is likely to choose a project A for investment because it guarantees high returns for the shareholders.
Conclusion
The table below summarizes the above computations of net present value, payback period, and return on investment.
Table 1.3 Summary of computations
From the table, it is apparent that project A has high net present value, a shorter payback period and a higher return on investment than project B. The results of the calculations are consistent.
This implies that there is no scenario where a project has a low net present value with a high return on investment. Therefore, the decision makers should choose project A because it is viable. Capital budgeting process is also necessary. This is because capital investments are irreversible. Expertise should be involved in making accept or reject decisions. This will help in eliminating the possibility of making wrong decisions.
Reference List
Brigham, F, & Ehrhardt, M 2010, Financial management theory and practice, Joe Sabatino, United States of America.
Dayananda, D, Irons, R, Harrison, S, Herbohn, J, & Rowland, P 2002, Capital budgeting: Financial appraisal of investment projects, The Press Syndicate of the University of Cambridge, United Kingdom.