Abstract
In this paper, we study the movement of a projectile – the physical phenomenon of the free flight of a body at a time when only the forces of gravity and resistance of a gaseous medium act on it. Within this area, the influence of the initial conditions of the projectile on the average speed of free flight before the first fall is checked, all other things being equal: body mass, initial velocity, and the angle of the beginning of a movement to the horizon. The data were obtained using an experiment in which bodies similar to the masses of projectiles were used, which were set in motion with their own hands, followed by recording the flight time and final distance, by which the average speed was measured.
Introduction and Background
The projectile motion along a curvilinear trajectory under gravity is described by the processes of classical mechanics and ballistics – the science of the motion of projectiles, mines, bullets, and unguided rockets when firing. Due to the object’s inertia, no external horizontal force is required to maintain the object’s horizontal velocity component (Mungan, 2018). When a projectile moves in the air, the power of air resistance acts on it in addition to gravity.
The magnitude of the force of air resistance, and hence the intensity of the impact on the projectile, can significantly exceed the force of gravity. This difference is more significant the less the weight of the projectile and the greater the speed of its flight (Yuliati & Mufti, 2020). At the same time, it should be noted that the value of the resistance force increases especially sharply when projectiles move at speeds exceeding the speed of sound.
Method
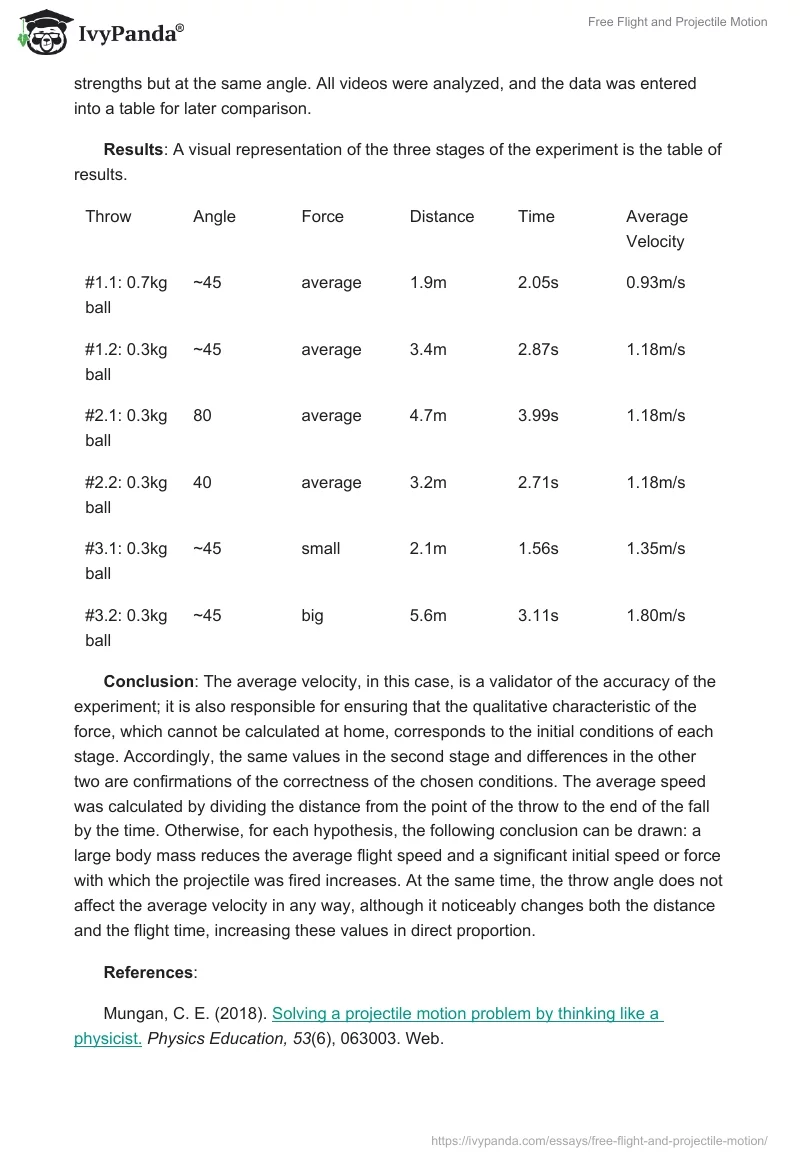
As part of the experiment, two balls were taken, one weighing 0.3 kg and the other approximately 0.7 kg. The action was divided into three stages, each consisting of two throws. Each throw was recorded on video to accurately estimate the time of flight and the moment of impact on the ground to fix the distance and the angle of the throw to the horizon. In the first case, body weight was evaluated as a factor affecting the projectile’s movement: two different balls were alternately thrown with the same force and angle.
In the second case, only a smaller ball was used, which was thrown at different angles to the horizon – the values are taken at approximately 80 and 40 degrees. Finally, the third stage included two throws of a small ball with different strengths but at the same angle. All videos were analyzed, and the data was entered into a table for later comparison.
Results
A visual representation of the three stages of the experiment is the table of results.
Conclusion
The average velocity, in this case, is a validator of the accuracy of the experiment; it is also responsible for ensuring that the qualitative characteristic of the force, which cannot be calculated at home, corresponds to the initial conditions of each stage. Accordingly, the same values in the second stage and differences in the other two are confirmations of the correctness of the chosen conditions.
The average speed was calculated by dividing the distance from the point of the throw to the end of the fall by the time. Otherwise, for each hypothesis, the following conclusion can be drawn: a large body mass reduces the average flight speed and a significant initial speed or force with which the projectile was fired increases. At the same time, the throw angle does not affect the average velocity in any way, although it noticeably changes both the distance and the flight time, increasing these values in direct proportion.
References
Mungan, C. E. (2018). Solving a projectile motion problem by thinking like a physicist.Physics Education, 53(6), 063003. Web.
Yuliati, L., & Mufti, N. (2020). Acquisition of projectile motion concepts on phenomenon-based physics’ experiential learning. In Journal of Physics: Conference Series (Vol. 1422, No. 1, p. 012007). IOP Publishing.