Introduction
The IS-LM model is based on the assumption that the general price level is fixed. This means that the general price level will not undergo sudden adjustments when economic conditions change.
Fiscal Policy in the IS-LM model
IS-LM model can be written using functional forms by focusing on the linear function. Considering the consumption function:
Where
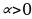is a constant and
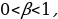is the Marginal Propensity to Consume (MPC), Y is the real income and T is the tax level,
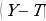is the disposable income. Investment function: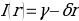
Where:
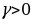is a constant and
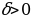measures the responsiveness of investment to the interest rate.
Government expenditure (G) is exogenous while tax level (T) is also exogenous. The equilibrium in the goods market is:
Using the functions we have above:
Solving for Y:
……………………. (i)
This is our IS curve. However the IS curve should have r as a function of Y.
Therefore making r the subject of the above IS curve equation we get:
…………………….. (ii)
Slope of the IS curve and the elasticity
When:
is particularly large the slope of the IS curve in (ii) is a small number in absolute value. Thus, a big change in income will result in a small change in r implying that the IS curve is relatively flat. In this case the IS curve is elastic with respect to Y.
On the other hand, if 
is particularly small, the slope of the IS curve in (ii) is a large number in absolute value. Thus, a small change in Y will result in a big change in r. The IS curve in (ii) will be relatively steep. In this case the IS curve is inelastic with respect to Y.
We define the money demand function as:
Where:
and 
are two parameters
The money supply 
and prices are exogenous. The equilibrium in the money market is given by:
The LM Curve is therefore given by:
……………………………. (iii)
Slope and elasticity of the LM curve
If h is large, the slope of (iii) is a small number and a big change in income will cause a small change in r. This implies that the LM curve is quite flat. Consequently, LM is “elastic” with respect to Y. If h is a small number, the slope of (iii) is a large number. The reverse of the aforementioned will occur resulting in an LM that is “inelastic” with respect to Y.
The IS-LM model is given by the following linear equations in the variables
and 
:
Solving this system produces the following solution:
………………………. (iv)
Where
………………………… (v)
Form the above functions we can see effects of the fiscal policy on the IS-LM model. The two variables that define a fiscal policy are G and T. From the equation (iv) above we have:
……………………….. (vi)
………………………… (vii)
An increase in G (ceteris puribus) will increase the equilibrium level of income while an increase in T (ceteris puribus) will decrease the equilibrium level of Y. From equation (v) above wee have:
…………………………. (viii)
…………………………… (ix)
An increase in G (ceteris puribus) will increase the equilibrium level of r while an increase in T (ceteris puribus) will decrease the equilibrium level of r. The increase in public expenditure (or a decrease in Taxes) increases the interest rate resulting in crowding out. Increase in government expenditure crowds out private investment.We can make the following conclusions:
- ΔG results in upward shift of the IS curve and increase of the real income by
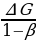. - Increase in G and Y also results in the increase of the interest rate due to increased money demand.
- An increase in r will decrease I thus partly offsetting initial increase in G. The equilibrium income will increase but by less than
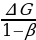, because of the crowding out effect.
Monetary policy in the IS-LM model
In the IS-LM model the only exogenous variable related to monetary policy is the level of money supply. So we consider a monetary policy as a change in the money supply. We can find the derivatives of equation (iv) and (v) to get:
……………………….. (x)
…………………………… (xi)
An increase in money supply (ceteris puribus) will increase the equilibrium level of output and decrease the equilibrium interest rate. An increase in M will shift the LM curve down, by an amount given by the change in M. For a given slope of the IS curve, the more the monetary policy is effective in affecting the level of real income, the less elastic is the LM curve. Given the slope of the LM curve, the reverse is true.
Weakness of the model
The model does not take care of the behavior of people and is only limited to a closed economy within the shorter. It is therefore an insufficient tool to predict or assess the behavior of the banks in an economy considering the long-term effects of some of these actions. The model can be modified to take care of long-term changes and shocks to the economy.
Bibliography
Cooke, Dudley. “IS-LM MODEL” Web.
Galí, Jordi , J. David López-Salido and Javier Vallés. “Understanding the Effects of Government Spending on Consumption,” Journal of the European Economic Association 2007 5, no.1(2007):227–270.