Abstract
The objective of the paper is to submit a five-section report of the M & M project. The first part focuses on sampling. The sample is made up of twenty-four bags of M&M candies. Each bag of M&M candy has six colors. These are blue, orange, green, yellow, red, and brown. Each bag of candy weighs 1.69 oz on average. These colors are recorded in an Excel workbook.
The second part of the project entails calculating the sample proportion of each color. In this section, a graph such as a bar graph or a histogram may be drawn to show the proportion of the various colors. The third and the fourth part entails coming up with confidence intervals for the percentage of the six colors. The fourth part entails testing claims of percentages of each color. The final part of the paper will entail writing a conclusion of the paper.
Introduction
This report is based on testing the hypothesis on the colors of M&M candies. The report examines the five parts of the M & M analysis. It also studies the method as a way of quality control of the production process. There is no well-defined approach used to collect data for the sample. The sample is collected randomly. This gives all elements in the population, even the chance of being selected. The parts of the paper comprise data collection, coming up with the proportion of the sample mean and sample proportion, confidence interval testing at 95%, and testing various claims. The objective of the paper is to provide an understanding of the packing of M&M candies using statistical methods (Larson & Farber, 2009).
Sampling
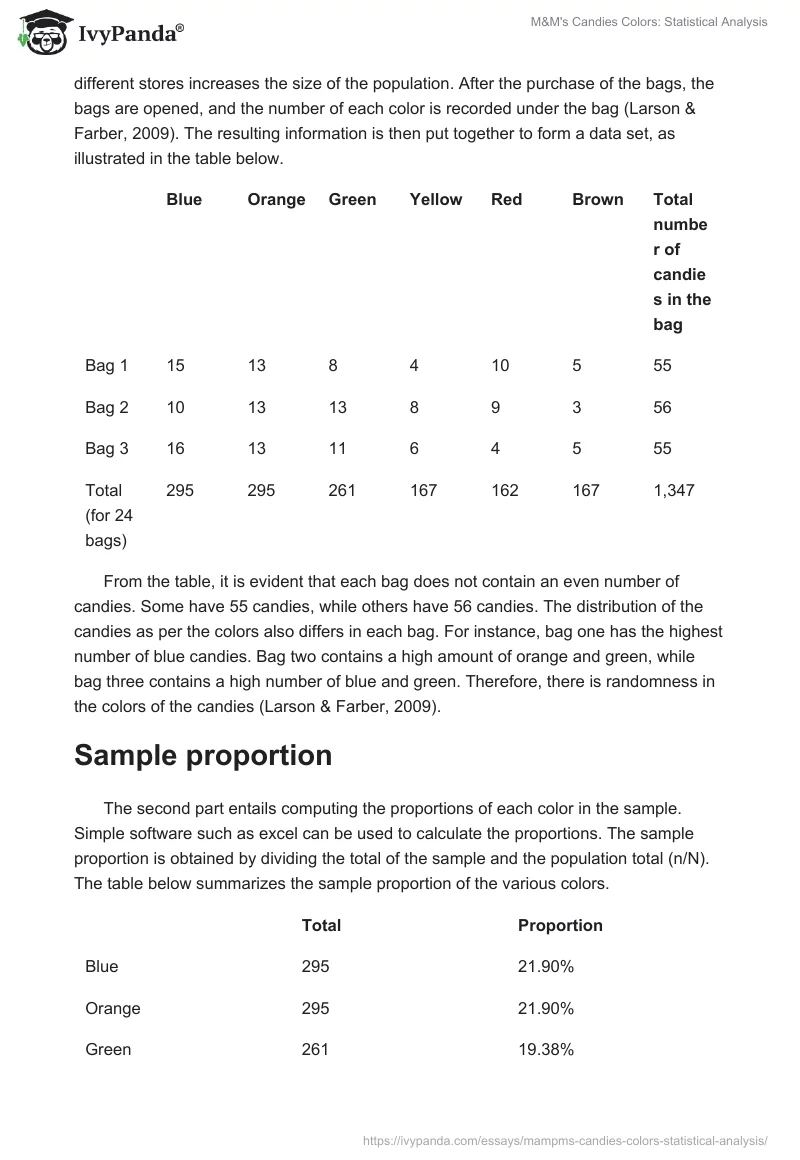
The first part of the project entails sampling. This part entails purchasing 24 bags of M&M candies. Each of the 24 bags weighs 1.69 oz. Further, the samples are purchased by different people from different outlets. The purchase of the candies from different stores increases the size of the population. After the purchase of the bags, the bags are opened, and the number of each color is recorded under the bag (Larson & Farber, 2009). The resulting information is then put together to form a data set, as illustrated in the table below.
From the table, it is evident that each bag does not contain an even number of candies. Some have 55 candies, while others have 56 candies. The distribution of the candies as per the colors also differs in each bag. For instance, bag one has the highest number of blue candies. Bag two contains a high amount of orange and green, while bag three contains a high number of blue and green. Therefore, there is randomness in the colors of the candies (Larson & Farber, 2009).
Sample Proportion
The second part entails computing the proportions of each color in the sample. Simple software such as Excel can be used to calculate the proportions. The sample proportion is obtained by dividing the total of the sample and the population total (n/N). The table below summarizes the sample proportion of the various colors.
From the calculations, 21.90% of the total population were blue, 21.90% were orange, 19.38% were green, 12.40% were yellow, 12.03% were red, and finally, 12.398% of the total population were brown. Also, from the proportions above, since the total number of candies is 1,347, it implies that the total number of blue candies is 295, the orange candies are 295, the green candies are 261, the yellow candies are 167, red candies are 162, and finally brown candies are 167.
The Sample Mean and Standard Deviation
Apart from the sample proportion, a sample mean is obtained for each bag. A sample standard deviation is computed from the sample mean calculated. The table below summarizes the sample mean and standard deviation for the various colors.
Further, it is observed that the average number of candies in a bag is 56.125, with a standard deviation of 1.5411. It is also evident that the mean number of blue balls is 12.29, with a standard deviation of 3.88, while the mean number of brown balls is 6.96, with a standard deviation of 3.32. Despite the large range of mean that is from 6.75 to 12.29, the standard deviation of the colors varies by a small range that is, from 2.34 to 3.88.
Confidence Interval at 95% Level of Confidence
This section entails computing the confidence interval for the various colors and the mean of the sample. The information on the confidence interval is summarized in the table below.
The confidence interval range is used to compute the upper and lower limits. The interpretation is that we are 95% confident that in a bag of candies, between 20.26% and 23.54% represent blue candies. The confidence interval for orange lies between 20.63% and 23.17%, for green lies between 17.86% and 20.9%, for yellow lies between 11.25% and 13.55%, for red lies between 11.04% and 13.03%, and brown lies between 10.98% and 13.78%. These intervals show the range of percentages of each color; one can pick from a random bag of candies. Also, we can deduce that at a 95% confidence interval, the mean number of candies in a bag will range between 55 and 56 (Healey, 2011).
Testing Claims
This section entails testing the claim that in a bag of M&M candies 24% of the candies are blue, 20% are orange, 16% of the candies are green, 14% of the total candies are yellow, 13% of the total candies are red, and 13% of the total candies are brown. The claims will be tested at a 5% level of confidence. The results for testing the claims are shown below.
Blue
From the above results, we do not reject the null hypothesis. This implies that there is no reason to doubt the claim of the company at the 5% level of confidence. This is because 24% falls within the confidence interval for blue, that is, 23.20% and 25.72%.
Orange
From the above results, we do not reject the null hypothesis. This implies that there is no reason to doubt the claim of the company at the 5% level of confidence. This is because 20% falls within the confidence interval for orange, that is, 19.74% and 22.12%.
Green
From the above results, we do not reject the null hypothesis. This implies that there is no reason to doubt the claim of the company at the 5% level of confidence. This is because 16% falls within the confidence interval for green, that is, 15.65% and 17.83%.
Yellow
From the above results, we do not reject the null hypothesis. This implies that there is no reason to doubt the claim of the company at the 5% level of confidence. This is because 14% falls within the confidence interval for the yellow color, which is 12.52% and 14.42%.
Red
From the above results, we reject the null hypothesis. This implies that there is reason to doubt the claim of the company at the 5% level of confidence. This is because 13% does not fall within the confidence interval for a red, which is 10.25% and 12.09%.
Brown
From the above results, we do not reject the null hypothesis. This implies that there is no reason to doubt the claim of the company at the 5% level of confidence. This is because 13% falls within the confidence interval for brown that is, 12.20% and 14.18%
Quality Control
From the above test of claims, red does not match the claims of the company. This can be as a result of over-packaging or under-packaging of red candies. This implies that there is a gap packaging of red candies. First, surveys should focus on the production process at the factory. This can be a poor working condition at the factory
Summary
The company does not have adequate evidence to support the claims of the number of red candies it packs. There is a possibility that either more or less red candies will be packed in a bag. However, there is sufficient evidence of the claims for percentages of the other colors. The company should investigate internal processes to ascertain where the problems arise (Larson & Farber, 2009).
References
Healey, J. (2011). Statistics: A tool for social research. USA: Cengage Learning.
Larson, R., & Farber, B. (2009). Elementary statistics: Picturing the world. New Jersey: Pearson Custom Publishing.