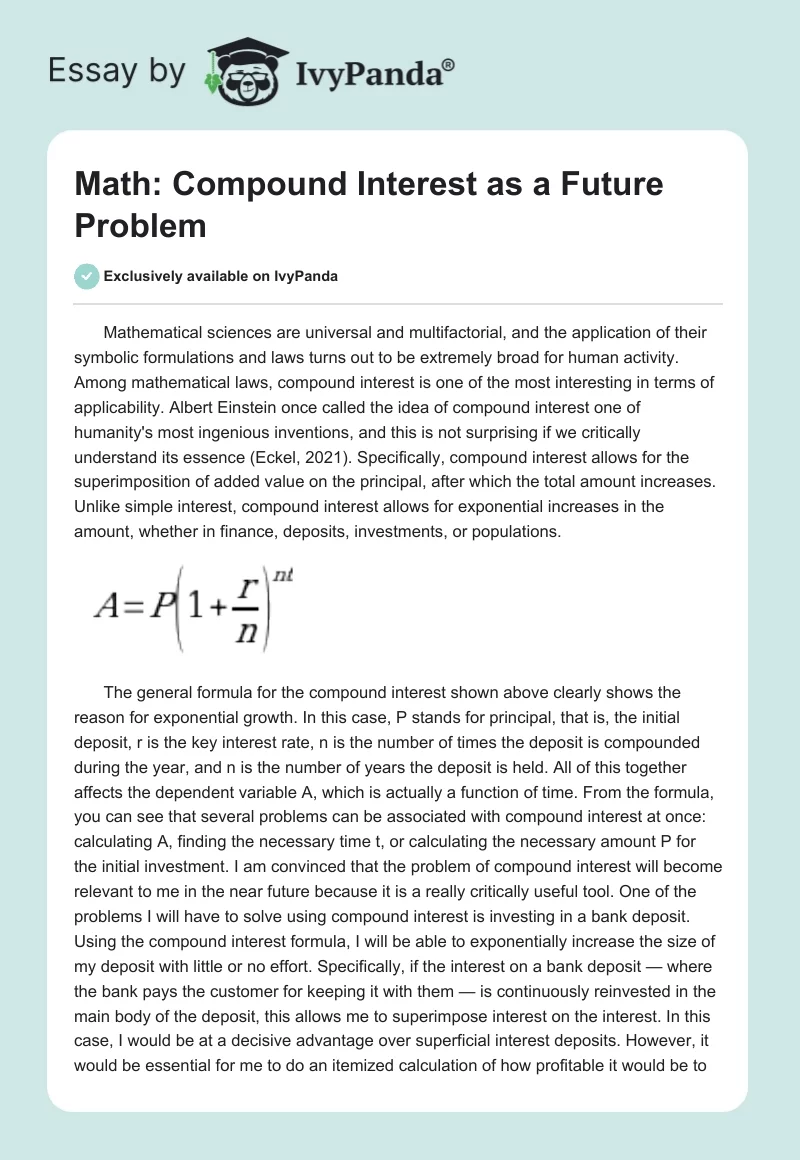
Mathematical sciences are universal and multifactorial, and the application of their symbolic formulations and laws turns out to be extremely broad for human activity. Among mathematical laws, compound interest is one of the most interesting in terms of applicability. Albert Einstein once called the idea of compound interest one of humanity’s most ingenious inventions, and this is not surprising if we critically understand its essence (Eckel, 2021). Specifically, compound interest allows for the superimposition of added value on the principal, after which the total amount increases. Unlike simple interest, compound interest allows for exponential increases in the amount, whether in finance, deposits, investments, or populations.
The general formula for the compound interest shown above clearly shows the reason for exponential growth. In this case, P stands for principal, that is, the initial deposit, r is the key interest rate, n is the number of times the deposit is compounded during the year, and n is the number of years the deposit is held. All of this together affects the dependent variable A, which is actually a function of time. From the formula, you can see that several problems can be associated with compound interest at once: calculating A, finding the necessary time t, or calculating the necessary amount P for the initial investment. I am convinced that the problem of compound interest will become relevant to me in the near future because it is a really critically useful tool. One of the problems I will have to solve using compound interest is investing in a bank deposit. Using the compound interest formula, I will be able to exponentially increase the size of my deposit with little or no effort. Specifically, if the interest on a bank deposit — where the bank pays the customer for keeping it with them — is continuously reinvested in the main body of the deposit, this allows me to superimpose interest on the interest. In this case, I would be at a decisive advantage over superficial interest deposits. However, it would be essential for me to do an itemized calculation of how profitable it would be to invest additional money in this deposit: for example, 10% of my stipend or salary each year. While it seems like it would increase profitability, it will be vital for me to see how relevant it is.
Meanwhile, I plan to take out a mortgage to buy real estate in the future, in which case compound interest may work against me. Specifically, if I do not pay back some of the money — minimum monthly payment — the bank will impose interest on the delinquency, which extends the body of the mortgage. As a financially literate person, I have to calculate my ability to cover at least the minimum payment so that compound interest does not work against me. Credit cards, which many people use, work roughly the same way. If I pay the bank back in full the amount I spent on the credit card, then I will be at zero balance. However, even one late or unpaid payment will cause my credit to increase, after which I will have to pay more interest.
An example should be given that clearly shows the calculation of compound interest, for example, for a bank deposit. If I decide to use $1,000 for a deposit, the most sensible way to invest it is with compound interest. Specifically, if I decide to use an annual compounding system at 5% per year, after three years, my $1,000 would turn into $1,157.63. At the same time, simple interest would only give me $1,150.00. After ten years, the difference would be more significant: $1,628.89 versus $1,500.00. For example, when I retire in fifty years, with compound interest, I could accumulate $11,467.40, which is about 3.3 times the profitability with simple interest.
Reference
Eckel, S. F. (2021). P5—the compound interest formula for professional development.American Journal of Health-System Pharmacy, 1-8. Web.