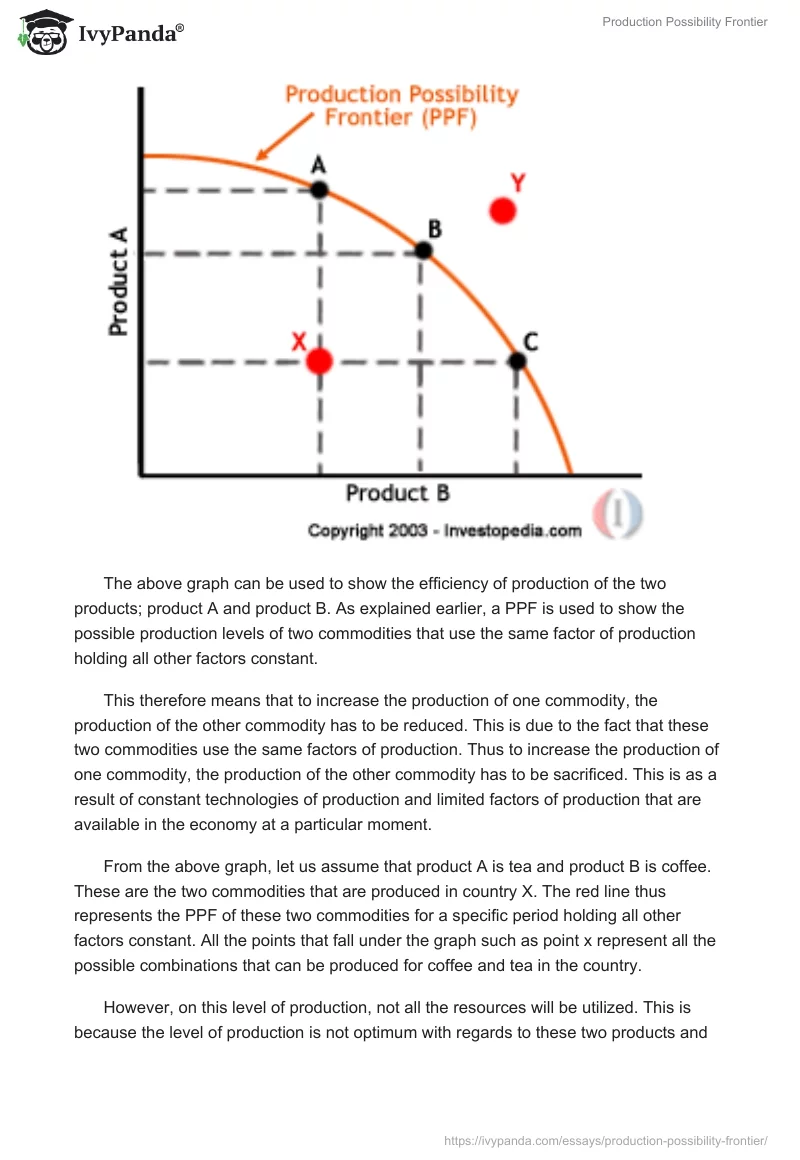
A production possibility frontier is a curve that represents the rates of production of two commodities that use the same factors of production within a specified period (Bradford, 2007). During this period, the technology that affects the process of production is assumed to be constant.
This ensures that the rates of production of these two commodities given the available factors of production remain constant. The production possibility frontier (PPF) thus shows the production levels of commodity A that results to a specific production level of commodity B. These commodities are either goods or services. In normal practice, the PPF curve has a concave curve that bulges downwards from the origin.
However, a straight line can also be used to represent the PPF. The shape of the PPF thus depends on the factors of production that are required to manufacture the goods or produce the services in question. A production possibility Frontier can be used to represent a number of economical factors. These include efficiency, opportunity cost, marginal rate of transformation and other economic indicators (Ramsey, 2007). The graph below represents an example of a PPF for commodity A and Commodity B.
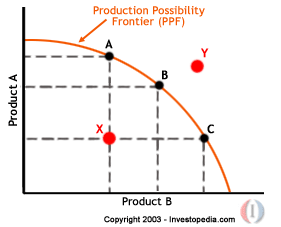
The above graph can be used to show the efficiency of production of the two products; product A and product B. As explained earlier, a PPF is used to show the possible production levels of two commodities that use the same factor of production holding all other factors constant.
This therefore means that to increase the production of one commodity, the production of the other commodity has to be reduced. This is due to the fact that these two commodities use the same factors of production. Thus to increase the production of one commodity, the production of the other commodity has to be sacrificed. This is as a result of constant technologies of production and limited factors of production that are available in the economy at a particular moment.
From the above graph, let us assume that product A is tea and product B is coffee. These are the two commodities that are produced in country X. The red line thus represents the PPF of these two commodities for a specific period holding all other factors constant. All the points that fall under the graph such as point x represent all the possible combinations that can be produced for coffee and tea in the country.
However, on this level of production, not all the resources will be utilized. This is because the level of production is not optimum with regards to these two products and the factors of production. Such a level of production is thus inefficient as some of the factors of production are not utilized (Turvey, 2005).
On the other hand, points of production above the PPF cannot be attained at the present moment with the use of the available factors of production and technology. Point Y is a good example of a level of production that country X cannot attain given its factors of production and level of technology at the present moment. However, such point may be attained in future if there are technological advancements and/or an increase in the factors of production for coffee and tea (Dreze, 2004).
Finally, the points along the curve represent the optimum level of production. This includes levels of production such as A, B and C. On these levels of production, all the factors of production are used to the maximum in the production of coffee and tea in country X. This is thus the most efficient level of production for country X.
From the graph, it can be seen that if production is set at point A, more quantities of tea (product A) will be produced and less quantities of coffee (product B will be produced). On the other hand, if production is set a point C, more quantities of coffee will be produced at the expense of tea. However, if production is set at point B equal amounts of these products will be produced. PPF can therefore be used to regulate the amount of production of these two commodities with regards to their demand in the market.
A PPF can also be used to measure opportunity cost. It has been stated that a PPF represents the possible combinations of production of two products given limited resources and factors of production.
Therefore, in the process of increasing the production of one commodity, some of the factors of production that were used to manufacture the other commodity will therefore have to be sacrificed. In the case of country X for example, if the government wants to increase the production of tea, it has to sacrifice the factors of production that were used to manufacture coffee.
As a result, the level of production of coffee will decline. In this respect therefore, the number of units of coffee that will be sacrificed to increase the production of tea represent the opportunity cost. Opportunity cost can be defined as the units of a commodity that are forgone to produce an extra unit of another commodity (Debreu, 2004). The above graph can therefore be used to calculate the opportunity cost of either of the two products.
PPFs are also used to calculate the marginal rate of transformation. Marginal rate of transformation is a term that is used to describe the amount of units of product A that have to be given up to produce an extra unit of commodity B (Bradford, 2007). The slope of the PPF at any point represents the marginal rate of transformation at that point.
At point A for example in the above graph, the marginal rate of transformation of product A can be calculated by simply calculating the slope of the curve at that specific point. The same method can be applied to calculate the marginal rate of transformation of product B at point C on the curve.
From the above discussion, it is evident that a PPF can be applied in various sectors of the economy. A household, a production company or a government to regulate the production of two goods that utilize the same factors of production can use a PPF. For efficiency to be achieved, production should be maintained at points within the curve.
However, the profitable point of production may vary along the curve. A firm should therefore produce at the point in which it earns maximum profits. This will be regarded as the optimum level of production of the firm. A PPF is thus a powerful tool for households, production companies and governments. This is because it enables them to determine the most efficient level of production to achieve maximum profits and meet the market demand.
References
Bradford, M. (2007). Optimal Departures from Marginal Costs System. American Economic Review, 6 (1), 15-22.
Debreu, F. (2004). Economics in the 21st Century. New York: Sage.
Dreze, C. (2004). Market Socialism. Boston: Long Horn Publishers.
Ramsey, L. (2007). Contribution to the Theory of Taxation. Chicago: Chicago University Press.
Turvey, T. (2005). Cost Benefit Analysis. Journal of Economics, 1 (5), 41-48.