The quantum hall effect is one of the fundamental discoveries that has made a profound effect on the field of condensed matter in physics (Girvin 3). When a two dimensional electronic system is subjected to a strong magnetic field at low temperatures, the system develops hall resistance with the electrons showing a plateau effect. Research shows that the quantum hall effect can occur at room temeperature, creating a condition known as room-temperature quantum hall effect in graphene. One of the practical areas of application include topological insulators. The focus of the study is on the effects of the hall effect on topological insulators at room temeperature.
Quantum hall effects at room temperature
According to Girvin, the phenomenon of the quantum hall effect at room temperature was discovered in 1980 and that has attracted much interest and research in that area (5). The study of the QHE room temperature that is known as room-quantum hall effect in graphene has helped to clarify and shed light into many issues in quantum physics.
Room temperature effects
One of the examples of QHE is the room-quantum hall effect in graphene that occurs at room temperature on a macroscopic scale. The findings created intense interest on the occurrence of the QHE at room temperature to clarify the behavior of the internal systems of materials. The results have led to the discovery of the resistance quantum in terms of h/e2 (where v is Planck’s constant and e is the charge on the electron) and the expression is used to define the properties of electron charges in a new metrological standard (Gusynin and Sharapov 7).
Tightly packed carbon atoms
It has been established that GHE observations can be made at exttremely low temperatures that are below the boiling point of helium gas and efforts to increase the temperature at which the observations can be made (at least 30 kelvin) using semi-conductors have been unsusccessful. However, the GHE effects can be observed at room temperature if tightly packed carbon particles are used for the QHE observations.
Graphene is one of the materials that have been successfully used to study the QHE at room temperature. Graphene “consists of tightly packed carbon atoms in a honeycomb forming a crystal lattice and each particle of the atoms are arranged in a lattice structure that form the honeycomb” (Gusynin and Sharapov 8). The sublattices create a topology that allows for the unusual energy dispersion of charge carriers in the topological structure (Gusynin and Sharapov 9).
Charge carriers
The rationale for the observations is that charge carriers on graphene, known as ‘Dirac fermions’ behave like the relativistic particles without mass. When the particles are subjected to ambient conditions, they move without being scattered. When the particles move, they create dirac points at the energy dispersion points near the charge neutrality area. The typical characteristics of the graphene material that makes it fit for the study is the unique property and behavior of charge carriers that are caused by the dispersion relationships of the fermions. According to Gusynin and Sharapov, ew speculations show that the entire lattice structure of graphene carbon substance can be used for the GHE study (12).
A experiment conducted to determine the behavior of the QHE of the graphene material used high mobility graphene samples that were deposited on silicon wafers using a simple but efficient extraction method to obtain mesoscopic graphite pieces. Afterwards, graphene samples measuring 3-10 μm were prepared and later placed in a Hall bar and an “oxygen plasma etching process was used to remove parts of the sample that were not required in the experiment (Gusynin and Sharapov 17). The entire experiment was set up and run to determine the results of the QHE on the graphene sample based on the magneto-resistance behaviour.
The results from the study showed that when the magnetic field was increased, an increase in the Zeeman spin splitting of the charge carriers were observed and the QH plateau at the Dirac point showed a finite increase. The results showed that a magneto resistance effect of the graphene material was detected in the experiment.
Effects on topological insulators
Scientists have shown signficant interest in the study of the hall effect that occurs at room temperatures on topological insulators. The study shows that the occurrence of the effects at room temperature is unique because most hall effects occur under extreme physical conditions. Evidence shows that the the behavior is based on spinning electrons that are caused by the effects of the internal magnetic field of the topological material being used. The behavior of the electrons of the topological materials is based on the laws of electromagnetism.
Here, a topological insualtor material is defined using the time ‘reversal symmetry’ at its surface. The topological material has characteristics that are similar to those of a good electronic conductor at the surface, but the interior of the topoligicla material has good insulating properties. It means that the surface of the material is a good conductor of electrons and the interior is a poor conductor of electorns. The properties that make the material special when compared with good conductors of electrons and insulators is that the topological insulator is symmetrically protected by the time reveral symmetry and the particle number conservation properties.
Electron band
Typcially, the electronic band of the non-interacting surface of the topological material is similar to that of an ordinary insulating material. Here, the Fermi level of electrons within the topological material lie between the valence and conductance electronic bands (Laughlin 23). The electrons on the the surface of the toplogical insulator acquire special states and that make them to move as charged particles. It enables the topological material to conduct electrons just like metals do because the electrons lie within the bulk energy gaps as shown in the diagram below.
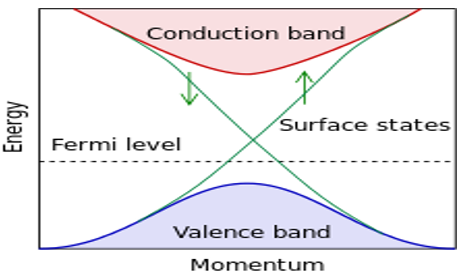
The surface state of the electrons is acquired when the particles spin in a momentum locking manner that is perpendicular to the direction of the momentum of the charged particles. Topological insulators have distinctive characteristics because of the edge state behavior of the particles that are caused by the ‘time reversal invariance’ and the ‘reverse and forward movement’ of the charged particles.
Conclusion
In conclusion, previous studies were not able to determine or make observations of the quantum hall effects on different materials because the effects occurred at extremely low temperatures and physical conditions. However, later studies show that the QHE is possible at room temperatures when using graphene and topological insulating materials. Observations provide ample opportunity for scientists to perform cost effective experements at room temperature on QHE effects.
Works Cited
Girvin, Steven M. The quantum Hall effect. No. cond-mat/9907002. 1999. Print.
Gusynin, V. P., and S. G. Sharapov. “Unconventional integer quantum Hall effect in.” Physical Review Letters 95.14 (2005): 146801.Print.
Laughlin, Robert B. “Anomalous quantum Hall effect: an incompressible quantum fluid with fractionally charged excitations.” Physical Review Letters 50.18 (1983): 1395-1398.Print.