The Demand for Food in South Africa Coursework

Abstract

Food consumption is an important issue in South Africa, given its relation to poverty and deprivation. With the pressing need to increase food security, understanding the determinants of the demand for food and having some estimates of the likely impact of price and income changes has become a vital task. There is, however, surprisingly little economic research on this topic and almost none in recent times. This paper provides a comprehensive empirical analysis of the demand for food in South Africa for the years 1970 to 2002. It moves beyond the usual static modelling approach in using a general dynamic log-linear demand equation and a dynamic version of the almost ideal demand system, to provide estimates of the short- and long-run price and expenditure demand elasticities.

Introduction

Studies of food consumption and expenditure have often been the subject of research in the developed and developing world. They provide important inputs into food and related nutritional policy initiatives, by providing estimates of how food consumption is likely to change with variations in prices, incomes and taxation. In the context of South Africa food policy is inextricably linked to food security. Despite its status as a lower-middle-income-country (World Bank, 2004), South Africa has considerable inequality and deprivation. It is estimated that about 35 per cent of the population in South Africa is vulnerable to food insecurity and approximately a quarter of the children under the age of 6 years are estimated to have had their growth stunted by malnutrition (Human Sciences Research Council, 2004, 3). Consequently, the Department of Agriculture devised ‘An Integrated Food Security Strategy for South Africa’ (IFSS) in July 2002. Food also has special significance, in the fight against HIV/AIDS. Nutrition is known to be important in determining the body’s ability to cope and ensuring those living with the disease lead extended, productive lives. Food also represents just under one third of consumer expenditure in South Africa, according to Reserve Bank estimates, declining from 35.6% in 1970 to 34.5% in 1990 and then to 28.4% in 2002. Thus there appears to be a relatively slow growth in real food expenditure, resulting in a consistent declining share of food spending. The question is whether the decline is a simple response to growing income, or is it being affected by relative price changes.

Understanding the demand for food is, therefore, a vital task, but there is surprisingly little economic research on this topic. The most recent time series studies on South African food expenditure are by Selvanathan and Selvanathan (2003) and Selvanathan and Selvanathan(2006). They use a demand system approach. Prior to that Balyamujura et al. (2000) replicate some earlier studies on South African food demand in order to project the impact of HIV/AIDS on the demand for food. Agbola et al. (2003) and the Human Sciences Research Council (2004) provide cross section studies. The studies find a range of results as, while they tend to start from a similar theoretical background, they differ markedly in the way they operationalise the models, the samples they use and the definitions of the variables.

In collecting the relevant data there are two possible definitions, the commonly used South African Reserve Bank category consisting of food, beverages, and tobacco and another more focussed food category which has data for a shorter period, also available from the South African Reserve Bank on request. Both are used in the empirical work and the results compared.

This paper investigates the determinants of food expenditure, taking the limited literature forward by providing a comprehensive time series analysis of the demand for food. It uses both a general dynamic log-linear demand equation and a demand system approach, estimating a dynamic form of the almost ideal demand system (Deaton and Muellbauer, 1980a and 1980b). These provide estimates of price and income elasticities in both the short run and the long run. In the next section, demand theory and the econometric approaches used in empirical work are briefly reviewed. Section 3 then considers the South African data, discussing the empirical models and the result of estimating them on the South African data. The elasticity estimates are summarised and discussed in section.

Finally, section 5 presents some conclusions and considers how the work could be developed in future.

Analysing the Demand for Food

There are two approaches that can be used to analyse the demand for food, the simple single demand equation method and the demand system approach1. Differences between the two are particularly apparent in the treatment of dynamics and so we use both. Starting with a demand function, it is specified as

qi = qi(p1, p2,… , pn, x) i = 1, 2, …, n. (1)

where qi and pi are the quantity demanded and price of the ith product, there are n products in total, and x = ∑_i p_i q_i is total expenditure. This general specification is operationalised as a log-linear demand equation:

ln qi = β₀ + β₁ ln pi + β₂ ln pj + β₃ ln pg + β₄ ln x + υ

Where ln indicates the natural log, qi is the quantity demanded of good i, pi is the price of good i, pj is the price of good j, pg is the general price level, and x is current total expenditure. To allow for dynamic analysis, lags of the dependent variable and explanatory variables are introduced.

An alternative to this approach is to estimate a system of demand equations2. The most popular system in the literature, due to its flexibility and ease of use is the almost ideal demand system of Deaton and Muellbauer (1980a and 1980b):

wi = αi + Σ_kδ k Dk + Σ j γ i j ln pj + βi ln (E/P)

where lnP = α0 + Σk αk ln pk + ½ Σj Σk γkj ln pj ln pj, E is total expenditure, wi = pi qi / E and the conditioning variables Dk can determine the intercepts of the demand functions. The system can be estimated equation by equation by using the approximation for ln P, ln P* = Σj wj ln pj. This is a static model but has been made dynamic in the literature by respecifying the theory (Dunne et al., 1984), or by specifying a general dynamic estimatable form of the regression (Anderson and Blundell, 1987; Smith, 1989). The latter approach is common in the literature. Recent studies which use dynamic forms of the almost ideal demand system, include Eakins and Gallagher (2003) on alcohol expenditure in Ireland, Duffy (2001) on food demand in the UK, Karagiannis et al. (2000) on the demand for meat in Greece. Song, Liu and Romilly (1997) use both an error correction model and an almost ideal demand system. For South Africa, Taljaard et al. (2004) focus on the demand for meat, using a dynamic almost ideal demand system, while Selvanathan and Selvanathan (2003) and Selvanathan and Selvanathan (2006) estimate a version of the Rotterdam demand system and Balyamujura et al. (2000) replicate some earlier studies on the demand for food using a single equation specification without dynamics in order to project the impact of HIV/AIDS on the demand for food3. The next section uses both a dynamic demand equation and the almost ideal demand system approach to analyse the demand for food in South Africa.

Empirical Results

Using the South African Reserve Bank database variable that defines food as ‘food, beverages and tobacco’, a general first order autoregressive distributed lag (ARDL) form of equation (1) is estimated:

Δ ln qt = β0 + β 1 Δ ln pft + β 2 Δ ln pgt + β 3 Δ ln x t + β 4 ln qt-1 + β 5 ln pft-1 + β 6 ln pgt-1+ β 7 ln x t-1 + ε t

There are four significant explanatory variables at the five per cent level or lower, and the diagnostic tests do not suggest any particular problems with the specification. The restrictions of short-run and long-run homogeneity imply that the short-run elasticities sum to zero and long-run elasticities sum to zero. This is imposed by introducing the relative price of food, rpft, and real total consumer expenditure, rx t, into the equation in place of the price of food and current total consumer expenditure:giving the results in Table 1.

Δ ln qt = τ₀+ α₂Δ ln rpft + α₃Δ ln rx t + α₁ ln q_t-1+ α₄ ln rpft-1+ α₅ ln rx t-1

Table 1. Results for dynamic demand equation, dependent variable, first difference of real food expenditure, Δ ln qt

The values in parentheses under the coefficients indicate t values, significant at the 1 percent level. ** significant at the 5 percent level. *** significant at the 10 percent level.

As the results in Table 2 show, all five explanatory variables are significant at the five per cent level or lower when these restrictions are imposed, with the only diagnostic test to suggest a problem being the Reset test for functional form. The F-test for the homogeneity restrictions indicate that they are not rejected.

A plot of the residuals suggested that 1972 might be an outlier and might be influencing the functional form (Reset) test result. There are three possible reasons why 1972 might have extreme values. Firstly, an exceptional rise in food prices, caused by severe cold spells in winter combined with drought conditions in summer, which accounted for 2 percentage points of the 7.4 per cent rise in consumer prices in 1972 (South African Reserve Bank Quarterly Bulletin, March 1973). Secondly, Rutter (2003) finding an extreme value for 1972, in a study of South African maize prices, suggested it was due to a deal between the US and Russia on the sale of wheat, which had a knock on effect on feed grain prices (Luttrell, 1973). Thirdly, the Foodstuffs, Cosmetics and Disinfectants (FCD) Act came into being in 1972 and could have led to an increase in the price of food if suppliers conforming to the regulations passed on any resulting costs to the consumer4.

Table 2. The dynamic demand model with homogeneity imposed, dependent variable, first difference of real food expenditure, Δ ln qt

The values in parentheses under the coefficients indicate t values, significant at the 1 percent level. ** significant at the 5 percent level. *** significant at the 10 percent level.

Introducing a dummy variable for 1972 to the error correction model with homogeneity imposed gives the results in Table 3. The dummy is significant at one per cent and its inclusion deals with the functional form issue. In addition, the homogeneity restrictions are still not rejected5.

This means that the short-run elasticities of demand with respect to the relative price of food and real total consumer expenditure are -0.6 and 0.5 respectively, while the values of the coefficients on the lagged levels suggest a long-run relation:

ln q = -1.24 ln rpf + 0.95 ln rx

Thus the long-run elasticities are -1.2 with respect to the relative price of food and 0.95 with respect to real total consumer expenditure. Testing for unitary elasticities by imposing them on the equation, one finds the price elasticity restriction is rejected, whereas the income elasticity restriction is not rejected6.

These results suggest that the demand for food is more responsive to price and income changes in the long run than in the short run. This would be expected for food as short-run consumption behaviour is likely to be affected by habit, whereas in the long run

Table 3. The dynamic demand model with homogeneity imposed and a 1972 dummy, dependent variable, first difference of real food expenditure, Δ ln qt

The values in parentheses under the coefficients indicate t valuesб significant at the 1 percent level. ** significant at the 5 percent level. *** significant at the 10 percent level.

consumers have time to adjust and change their pattern of consumption. The fact that the long-run income elasticity result is nearly unity could be because of the high proportion of poor households in the South African population. Increased incomes are matched by a proportionate rise in expenditure on food in the long run. Certainly the results estimated by Selvanathan and Selvanathan (2006) indicate that income elasticities of developed countries are on the whole lower than those of developing countries; the authors incorporate South Africa in the latter category7.

The long-run price elasticity of -1.2 is a concern, as it is much larger than one might expect for a necessity. This might arise from the definition of food in the data. In fact the category is ‘food, beverages and tobacco’. While this is in common use, it could be argued that the constituent components act differently. Thus, when there is an increase in the price of only food, the consumption of tobacco and beverages, may be reduced fairly substantially to ensure basic food needs are met. On the other hand the addictive nature of tobacco and alcoholic beverages might limit such adjustments in practise.

It is possible to obtain a less broadly defined food variable from the South African Reserve Bank on request, however only for a shorter period 1976-2002. Using this ‘only food’ variable as the dependent variable with homogeneity imposed gives:

ln qfot =γ₀ + γ₂Δ ln rpfot + γ₃Δ ln rx t + γ₁ ln qfot-1 + γ₄ ln rpfot – 1 + γ₅ ln rx t -1 + ut

The results are shown in Table 4, suggesting a relatively well specified model, for which the homogeneity restriction is not rejected.

Table 4. The dynamic demand model with homogeneity imposed: ‘only food’, dependent variable, first difference of real ‘only food’ expenditure, ln Δ qfot

The values in parentheses under the coefficients indicate t valuesб significant at the 1 percent level. ** significant at the 5 percent level. *** significant at the 10 percent level.

With the more restricted definition of food, the price elasticity is indeed lower, with the short-run price elasticity -0.5. The short-run the income/expenditure elasticity remains unchanged at 0.5. The long-run relationship is:

ln qfo = -0.77 ln rpfo + 0.63 ln rx

implying a long-run price elasticity of -0.8, though this is based on an insignificant coefficient for the relative price, and an expenditure elasticity of 0.6.

These results do seem to support our concerns, as excluding beverages and tobacco from the variable does reduce the elasticities. However, the differences in the short-run elasticities are not as marked as the differences in the long-run elasticities. The income elasticity estimates of both regressions show food to be a normal good as its demand increases as income increases, and it is income inelastic in both the short run and long run. Such results meet the expectations for a necessity. The ‘only food’ analysis suggests that own price is not an important variable in the long run, whereas the broad food category indicates that own price is significant in the long run, and it has an elastic demand8. Using the almost ideal demand system, equation (3), and introducing dynamics, following Smith (1989), gives equations for food and non-food:

Δ sqt = a₁₀+ δ₁₁Δ ln pft + δ ₁₂Δ ln pnft + β₁₁Δ ln ( x – P*)t + λ₁₁ sqt-1 + δ₁₃ ln pft-1

+ δ₁₄ ln pnft-1 + β₁₂ ln ( x/P*) t-1 + ε₁ (7)

Δ snfqt = a ₂₀ + δ₂₁Δ ln pft + δ₂₂Δ ln pnft + β₂₁Δ ln ( x – P*)t + λ₂₁ snfq t-1 + δ₂₃ ln pft-1 +

δ₂₄ ln pnft-1 + δ₂₂ ln ( x/P*) t-1 + ε₂ (8)

Where, sqt is the share of expenditure on food and snfqt, the share of non-food expenditure. The natural log is indicated by ln, pft is the price of food, pnft is the non-food price and ( x/P*) is consumption expenditure in current prices divided by an index of prices, P* 9. Note that the dependent variables do not sum to one, as the dynamic form is not a singular system.

Table 5: Dynamic almost ideal demand system: dependent variable, first difference of the share of food, Δ sqt

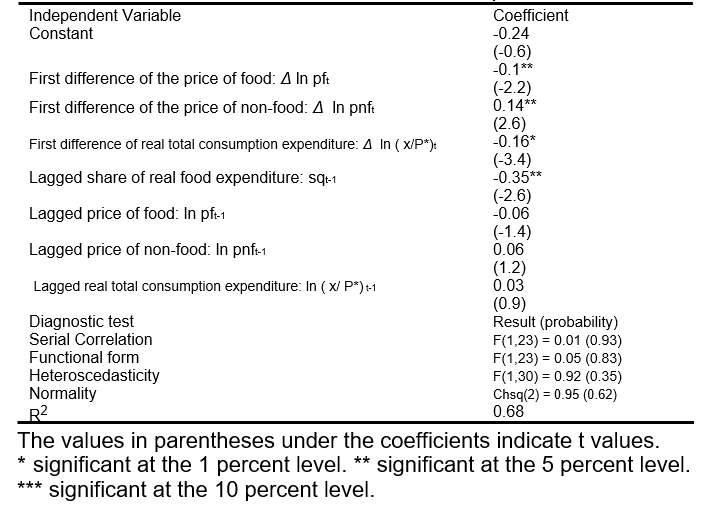
The estimation results are presented in Table 5 and show all first difference terms and the lagged dependent variable to be significant at the five per cent level or lower, but the lagged levels coefficients are all insignificant. The results for the diagnostic tests suggest no particular problems with the specification of the model.

Imposing homogeneity on the system implies using the relative price of food, so taking:

ln pfft = Δ ln pft – Δ ln pnft and ln pfft-1 = ln pft-1 – ln pnft-1 gives for food:

Estimating this equation gives the results in Table 6 and now all the independent variables are significant at the five per cent level or lower, with the exception of lagged real total consumption expenditure. Homogeneity is not rejected and the diagnostic tests do not show any evidence of misspecification.

Table 6. Dynamic almost ideal demand system with homogeneity imposed: dependent variable, first difference of the share of food, Δ sqt

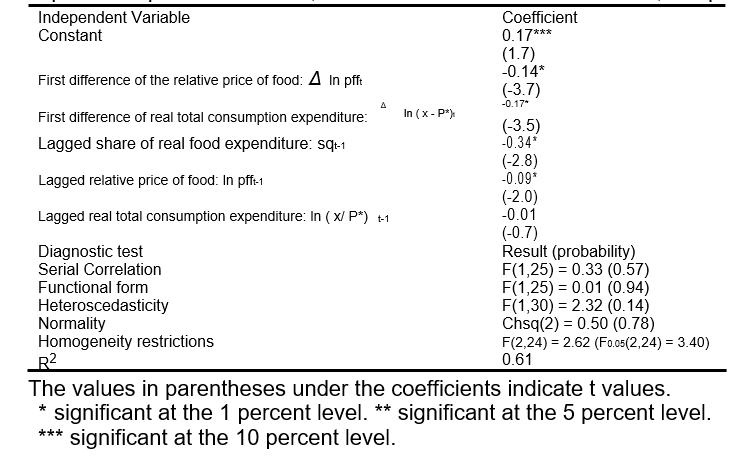
Within the demand system the coefficients are not elasticities, they are measuring the response of shares to changes in price and income, though a negative sign on the income/expenditure coefficient does indicate that food is a necessity (Deaton and Muellbauer, 1980b)10. Computing the short-run elasticities gives a value of 0.5 for income elasticity and 0 for the compensated price elasticity, with the latter being rather different to that calculated using the single equation log-linear model. The long-run elasticities are 1.0 for income, as expected given the insignificance of the total real expenditure term, and -0.9 for the price elasticity.

Estimating the almost ideal demand system model for ‘only food’ gives the results shown in Table 7. The homogeneity restriction was not rejected. It shows that the coefficient on the first difference real expenditure term is not significant at the five per cent level or less. The lagged level of real expenditure is significant at six per cent. All the other regressors are significant at one per cent, apart from real expenditure, which is insignificant, and there are no obvious problems with the specification.

Table 7. Dynamic almost ideal demand system with homogeneity imposed: dependent variable, first difference of the share of ‘only food’

The values in parentheses under the coefficients indicate t valuesб significant at the 1 percent level. ** significant at the 5 percent level. *** significant at the 10 percent level.

Contrary to expectations, the move to the more restrictive category of food does not reduce the price coefficients and elasticities as it tends to do in the single equation models. The insignificance of both real expenditure terms suggests unitary income elasticities in the short and long run, while the price elasticities estimates are -1.2 for the short run and -1.3 for the long run. The next section brings together and discusses the results of all the regressions.

Elasticity Estimates

Table 8 presents the elasticity estimates found in the empirical analysis. Looking at food, beverages and tobacco results, the single equation and system methods give similar estimates for the income elasticities. They are 0.5 for the short run and 1.0 for the long run. They differ in their price elasticity estimates, though both methods show the short-run values to be lower than the long-run values. The demand system gives smaller price elasticities than the single equation estimation. In the short run the price elasticity is equal to zero for the system, whereas this elasticity is -0.6 for the single equation regression. The long-run price elasticities are closer for the two methods, with the demand equation just over one and the demand system just under one. Using ‘only food’, at the cost of degrees of freedom, tends to give smaller long-run elasticities for the demand equation in comparison to the food, beverages and tobacco equation. Such a result is anticipated. However, the same conclusion does not apply when comparing the ‘only food’ system with the food, beverages and tobacco system.

Table 8. Elasticity Estimates

Demand equation: Food, beverages and tobacco

Demand equation: Only food

Demand system: Food, beverages and tobacco

Demand system: Only food

The results indicate that the system approach does not resolve the anomalies of the single equation method. However, while caveats exist, the results do allow some conclusions to be drawn. Examining the long-run elasticities, food, beverages and tobacco would appear to be price elastic, although the estimate is -0.9 for the system, suggesting that an increase in price will lead to a more than proportionate decline in demand. Food, the more restrictive category, is price inelastic, though close to one in the long run, for the demand equation, but price elastic for the system. Food, beverages and tobacco seem to have unit income elasticity in the long run, implying that this category of expenditure will increase proportionately with income. Food alone, again produces different results for each method. The equation regression shows food to be income inelastic, and for the system, the income elasticity is unity. Turning to the short-run elasticity estimates, these estimates are also not completely consistent across the two estimation approaches, but both income and price elasticities are generally smaller in the short run than the long run. Food, beverages and tobacco would seem to be price and income inelastic, while ‘only food’ is also price and income inelastic for the demand equation, but it is price elastic for the system, with almost unit income elasticity.

Considering other recent estimates, in Table 9, the Selvanathan and Selvanathan (2003) consumer demand system study, 1960-2001, gives an estimate for the price elasticity of food, broadly defined, of -0.32 and for income elasticity of food, their estimate is 0.81. More recently, Selvanathan and Selvanathan (2006) have separated out food from beverages and tobacco, and employing the same system approach as in their earlier paper, they obtain a price elasticity of demand for food of -0.43 and an income elasticity of demand for food of 0.66 for South Africa, one of forty-three countries studied. This result is interesting as the price elasticity estimate increases for ‘only food’, but the income elasticity falls. Balyamujura et al. (2000), using a single equation model of demand described earlier in the paper, estimate that the price elasticity of demand, in terms of the consumer price index, is -0.90 and the income elasticity of the demand for food is 0.58. Other earlier studies generally found lower estimates.

Table 9. A summary of earlier studies on elasticity estimates for food demand in South Africa

While care must be taken in comparing estimates across different time periods and different studies, the later studies do seem to be reporting higher estimates for the food elasticities (larger negative in the case of prices). Our results provide even higher estimates, suggesting there may be an increasing sensitivity of food demand to changes in prices and income.

Conclusion

This paper has provided an empirical analysis of the demand for food in South Africa for the period 1970 – 2002. Rather than focussing on one particular method it has taken an ‘ad hoc’ approach, estimating a general dynamic log-linear demand equation, as well as a ‘demand system’ approach, estimating a dynamic form of the almost ideal demand system. These have both provided estimates of long- and short-run price and income elasticities, but they are not wholly consistent. Further, concerns with the broad but commonly used definition of food, which includes beverages and tobacco, have led to estimates being made for a more restrictive definition of the food variable, though this had been at the expense of degrees of freedom.

The empirical analysis has allowed us to make some tentative conclusions, but possibly more importantly provides a warning of the need to consider a range of approaches and studies if feeding the results into policy recommendations. Previous studies often have a similar theoretical background, but differ noticeably in the way they operationalise their models; some employ systems of equations, others use single equation estimation, some do not go beyond static models and others introduce dynamic models. Together with possible different definitions of the data and lengths of time series, it is not surprising that a range of results has been found.

With caveats, the results do allow some conclusions to be drawn. Food would seem to be increasingly elastic both in price and income compare to previous periods. Food, beverages and tobacco would appear to have a relatively high price elasticity tending toward a price elastic estimate in the long run, suggesting that an increase in price will lead to almost the same or a more than proportionate decline in demand. The food, beverages and tobacco category also seems to have unit income elasticity in the long run, implying that it will increase proportionately with income. For the more restrictive category, ‘only food’, the results suggest that long-term price elasticity is close to unit elasticity or higher. With regard to income elasticity, ‘only food’ is either inelastic or unit elastic in the long run. The single equation estimates suggest that the more restrictive category ‘only food’ is characterised by less elastic long-run price and income elasticities.

The short-run elasticity estimates were not completely consistent across the two estimation approaches, but were generally smaller than the long run ones. Examining the estimates of both approaches, food, beverages and tobacco would seem to be generally price and income inelastic, while for food alone the results veer between price inelastic and price elastic, with almost unit income elasticity. Comparing these results with earlier studies the elasticities are considerably higher.

Information on elasticity estimates can provide policy makers with an indication of how South African consumers react to changes in the price of food, taxes and changes in income. Such changes may arise directly through food and nutrition polices, or from adjustments within the economy, whether it is the growth of the economy or, for example, some adverse shock to the agriculture sector. The size of the elasticities we have estimated should be a cause for concern to policymakers.

This paper has made a contribution towards understanding the demand for food, it also acts as a warning of the care needed to undertake and interpret empirical work on the subject. It illustrates the importance of using a range of empirical methods before drawing conclusions and the need to be wary of taking the results of any particular empirical analysis as the basis for policy. The study does have limitations. It uses aggregate data with aggregated categories of food and while this has allowed a consistent time series analysis, it cannot capture consumer adjustments in their choice of foodstuffs, or in their spending on other essential goods and services11. Future work will need to operate at a more detailed level, if data becomes available and consider changes in household food expenditure composition. Household level studies can provide further understanding of the processes at work, particularly if they are undertaken with panel data.

References

Agbola, F., W, Maitra, P and Mclaren, K. (2003) On the Estimation of Demand Systems With Large Number of Goods: An Application to South Africa Household Food Demand. 41st Annual Conference of the Agricultural Economic Association of South Africa (Aeasa).

Anderson, G. And Blundell, R. (1982) “Estimation and Hypothesis Testing in Dynamic Singular Equation Systems”, Econometrica, 50: 1559-76.

Balyamujura, H., Jooste, A., Van Schalkwyck, H. And Carstens, J. (2000) Impact of the HIV/Aids Pandemic on the Demand for Food in South Africa. Presented at the Demographic Impact of HIV/Aids in South Africa and Its Provinces, East Cape Training Centre (Etc Conference Centre), Port Elizabeth.

Barr, G.D.I. Estimating a Consumer Demand Function for South Africa. South African Journal of Economics, 51(4): 523 – 529.

Contogiannis, E. (1982) Consumer Demand Functions in South Africa: An Application of the Houthakker and Taylor Model. South African Journal of Economics, 50(2): 125-135.

Deaton, A. And Muellbauer, J (1980a) An Almost Ideal Demand System, American Economic Review, 70 (3): 312-26. (Classic Article Essential Reading.)

Deaton, A. And Muellbauer, J. (1980b) Economics and Consumer Behaviour. Cambridge: Cambridge University Press.

Department of Agriculture, Republic of South Africa. (2002) The Integrated Food Security Strategy for South Africa. Department Agriculture: Pretoria.

Dockel, J.a. And Groenewald, J.a. (1970) The Demand for Food in South Africa. Agrekon, 9 (4): 15 – 28,

Duffy, M. A. (2001) Cointegrating Demand System for Food in the United Kingdom. Working Paper Series 0108, Manchester School of Management, Umist.

Dunne, J.p., Pashardes, P. And Smith, R.p. (1984) Needs, Costs, and Bureaucracy: The Allocation of Public Consumption in the UK Economic Journal, 94: 1-15.

Dunne, J.p. And Smith, R.p. (1983) “The Allocative Efficiency of Government Expenditure: Some Comparative Tests”, European Economic Review, 20: 381-394.

Eakins, J.M. And Gallagher, L.a. (2003) Dynamic Almost Ideal Demand Systems: An Empirical Analysis of Alcohol Expenditure. Applied Economics, 35: 1025-1036.

Human Sciences Research Council, Integrated Rural and Regional Development. (2004) Food Security in South Africa: Key Policy Issues for the Medium Term. Web.

Karagiannis, G., Katranidis, S. And Velentzas, K.( 2000) An Error Correction Almost Ideal Demand System for Meat in Greece. Agricultural Economics, 22: 29-35.

Luttrell, C. B. (1973) The Russian Wheat Deal – Hindsight vs Foresight. Federal Reserved Bank of St Louis ,1973, 2-9. Web.

Rutter, J. (2003) Model Development for South African Producer Price Maize (1970-2001). Unpublished Research Paper, University of Tennessee, 2003. Web.

Selvanathan, E.a. And Selvanathan, S. (2003) “Consumer Demand in South Africa.” South African Journal of Economics, 71(2):325- 344.

Selvanathan, E.a. And Selvanathan, S (2006) Consumption Patterns of Food, Tobacco and Beverages: A Cross-Country Analysis. Applied Economics, 38: 1567 – 1584.

Smith, R.p. (1989) Models of Military Expenditure, Journal of Applied Econometrics, 4: 345-359.

Song, H., Xiaming, L. And Romilly, P. (1997) A Comparative of Modelling the Demand for Food in the United States and the Netherlands. Journal of Applied Econometrics,12(5): Special Issue: The Experiment in Applied Econometrics, 593 – 608.

Southern Africa Labour and Development Research Unit (Saldru) (1993) South African Integrated Household Survey (Sihs). Web.

South African Market of Food and Beverages. Durban, South Africa, 1997. Itc Projects Raf/24/70 & Raf/47/51. Web.

South African Reserve Bank (Various Years) Quarterly Bulletin. South African Reserve Bank, Pretoria.

South African Reserve Bank. Web.

Taljaard, P.r., Alemu, Z.g. And Van Schalkwyk, H.D. (2004) The Demand for Meat in South Africa: An Almost Ideal Estimation. Agrekon, 43(4), 430 – 443.

Thomas, R.L. (1997) Introductory Econometrics: Theory and Applications. 2nd Edition. Harlow: Longman Group, UK Limited.

World Bank, 2004.