The exponential function is essential in analytic algebra and is widely used in all related fields. It is unknown when man first began using exponential laws, but the first documented appearance of the exponent in printed works dates back to 1544. Exponents were written about in the book Arithmetic Integra by the German monk Michael Stifel (Reich, 2017). However, it is essential to say that Stifel did not use other bases for exponentiation than two, which means that there was no real exponentiation on the part of the German. Exponents are thought to have been found in the Sumerian language of ancient Babylon as well (Smith, 2017). However, because of the qualitative difference in the use of the mathematical alphabet, the Babylonian exponentiation was different from what is used today.
This knowledge helps paramountly to know that exponentiation is not only the raising of a number to a degree but also the historical practice of working with numbers that communities have tried to come to in the course of development. It gives insight into how people worked with multiplication operations on the same number before they even knew what an exponent was. However, today’s students know these concepts perfectly well, and this contrast could be used to teach them effectively. For example, an appropriate pedagogical practice would be for a modern schoolboy to “dialogue” with a primitive man to explain what in the “future” is called exponentiation.
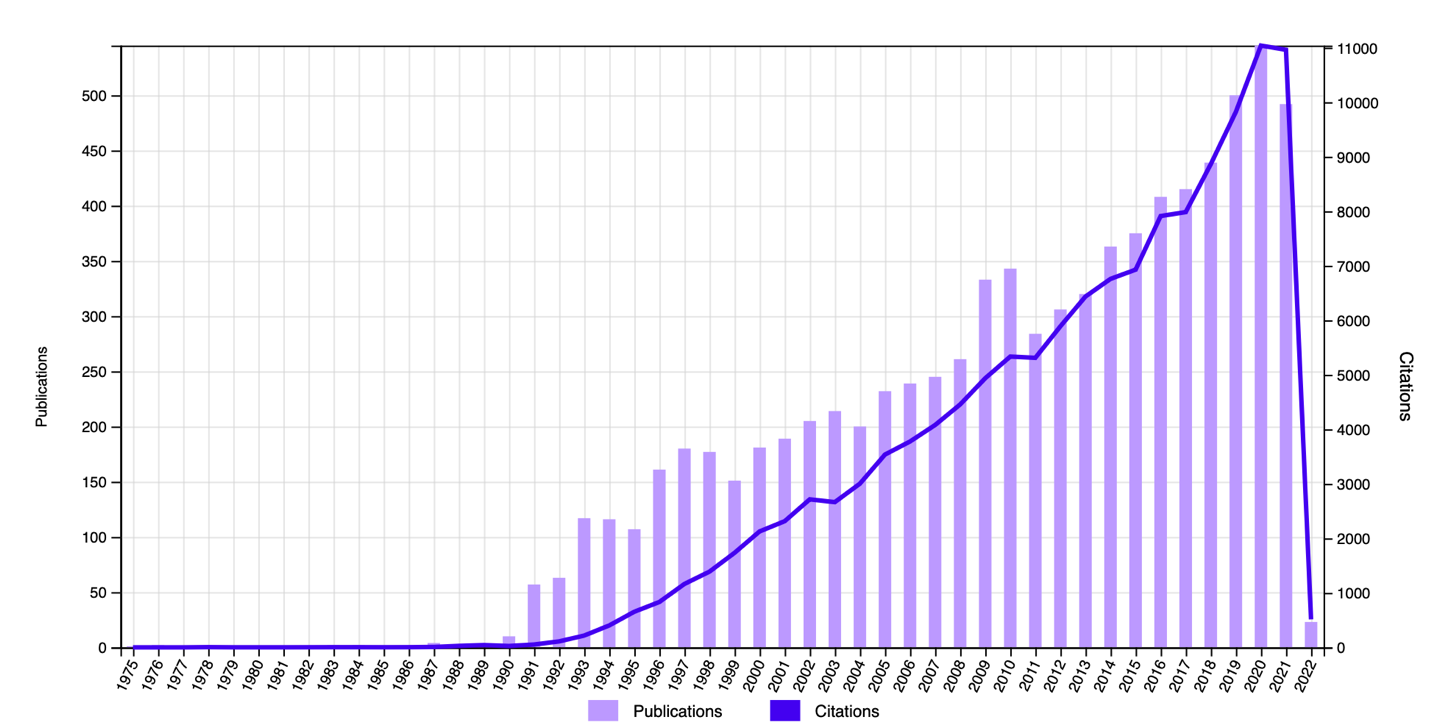
Meanwhile, functional analytic algebra had hardly evolved in previous centuries, and even the use of exponents was not associated with a graphical reflection of the patterns being studied. Exploring the contributions of different eras to the universal knowledge of exponents can be an interesting practice for students, reflecting the retrospective and continuity of mathematics. In this sense, the teacher might ask students to collect information on the number of scientific papers published each year over the past five decades. Plotting this data on the Cartesian plane will produce an almost exponential growth in the number of papers published, an example of which is shown in the Figure below. This practice will help the child track not only the expansion of the scientific community over time but also the application of the exponential law.
References
Reich, U. (2017). Mathematik. In G. Frank (Ed.), Philipp Melanchthon (pp. 559-576). De Gruyter.
Smith, R. C. (2017). Exponents, history, civilization. Tracing Curves. Web.