Most, if not all people will agree that time is critical in determining the actual value of money. Often you would hear people complaining that what $100 could buy 5 years ago, cannot even be bought with $90 today. The same is true today. It would be better to have $200 today than have the same a year later. Simply put, the same value of money today is worth the same value in future. The time value of money can therefore be defined as the calculated value of the money taking into consideration various time aspects that affect its value.
Financial managers are crucial to everyday operations of organizations and as such, it is important that they understand the time value of money. This understanding forms an important aspect of planning for the future expenses. Knowing what value a given amount of money is expected to return after a specified period of time is useful in developing the entities financial projections.
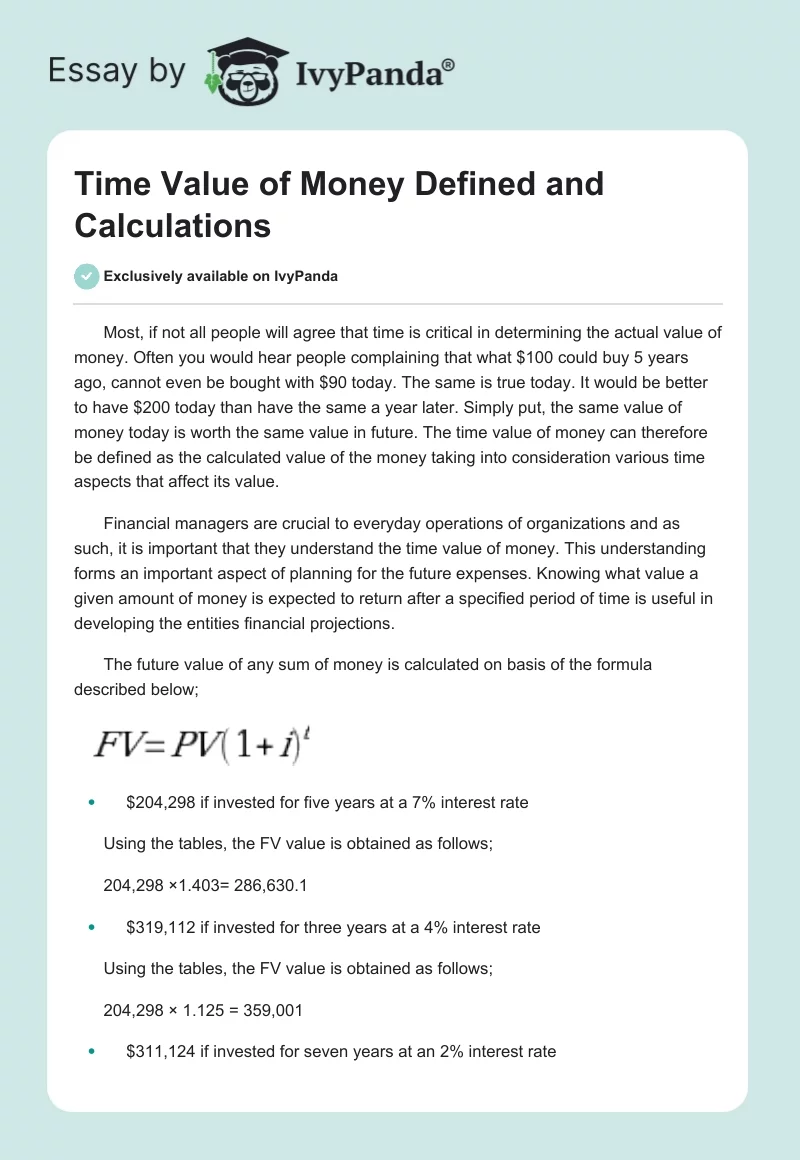
The future value of any sum of money is calculated on basis of the formula described below;
- $204,298 if invested for five years at a 7% interest rate
Using the tables, the FV value is obtained as follows;
204,298 ×1.403= 286,630.1
- $319,112 if invested for three years at a 4% interest rate
Using the tables, the FV value is obtained as follows;
204,298 × 1.125 = 359,001
- $311,124 if invested for seven years at an 2% interest rate
Using the tables, the FV value is obtained as follows;
311,124 × 1.149 = 357481.476
- $299,129 if invested for ten years with a 0.9% interest rate
Using the tables, the FV value is obtained as follows;
299.129 × 1.094 = 340286.8574
Calculate the present value of the followings
The present value is obtained by the equation provided below;
The calculations provided below are based on the PV tables,
- $652,126 to be received three years from now with a 4% Interest rate = $ 652.126 × 0.889 = $ 579740.014
- $128,231 to be received five years from now with a 5% interest rate = $ 128231 × 0.784
- $591,199 to received two years from now with a 12% interest rate = $ 591185.603 × $ 471185.603
- $187,111 to be received eight years from now with a 1% interest rate = $187111 × 0.923 = $ 1727035.453
Suppose you are to receive a stream of annual payments (also called an “annuity”) of $193,723 every year for three years starting this year. The interest rate is 4%. What is the present value of these three payments?
Based on the PV annuity tables, the present value of the three payments is as shown below;
$ 193723 × 2.775 = $ 537581.325
Suppose you are to receive a payment of $292,595 every year for three years. You are depositing these payments in a bank account that pays 2% interest. Given these three payments and this interest rate, how much will be in your bank account in three years?
Based on the table of future value (annuity), the future value of the payments is as shown below,
$ 292.595 × 3.06040 = $ 895457.738
The calculations as well as the theoretical knowledge covered in the previous questions offer not just an avenue for understanding calculations involving time value of money but also provides an in-depth understanding of the importance of creating a distinction between the present value of money and the value of the same at a later time. Additionally, they help one understand what a value they are expecting in future is at the present time.