Introduction
A particle object responds to an external force by remaining in its original orientation. Any object that may be rotated by force is considered an extended object. Furthermore, this experiment will see that the force can alter an object’s equilibrium (Camley & Brown, 2019). Torque is the force that causes an object to rotate in a circle around its axis. In theory, torque is the act of turning an item over. Any rotational acceleration applied to an object will be due to the net torque acting on it. According to Feng et al. (2022), torque is proportional to the product of the applied force’s magnitude and its perpendicular Distance from the axis of rotation, which in turn is determined by the direction of the force and the angular separation between the two. If the application of forces causes the object to move but not rotate, it behaves like a point particle.
Objective and Purpose of the Experiment
The torque on a thin rod experiment was to gather information on the forces acting on the rod so that mathematical models could be created to depict the connection between the independent and dependent variables. This experiment aimed to gather data on the forces acting on a thin rod while it was balanced so that graphical and mathematical models of the system could be constructed. The connections between dependent and independent variables were modeled using these representations.
The Procedure of the Experiment
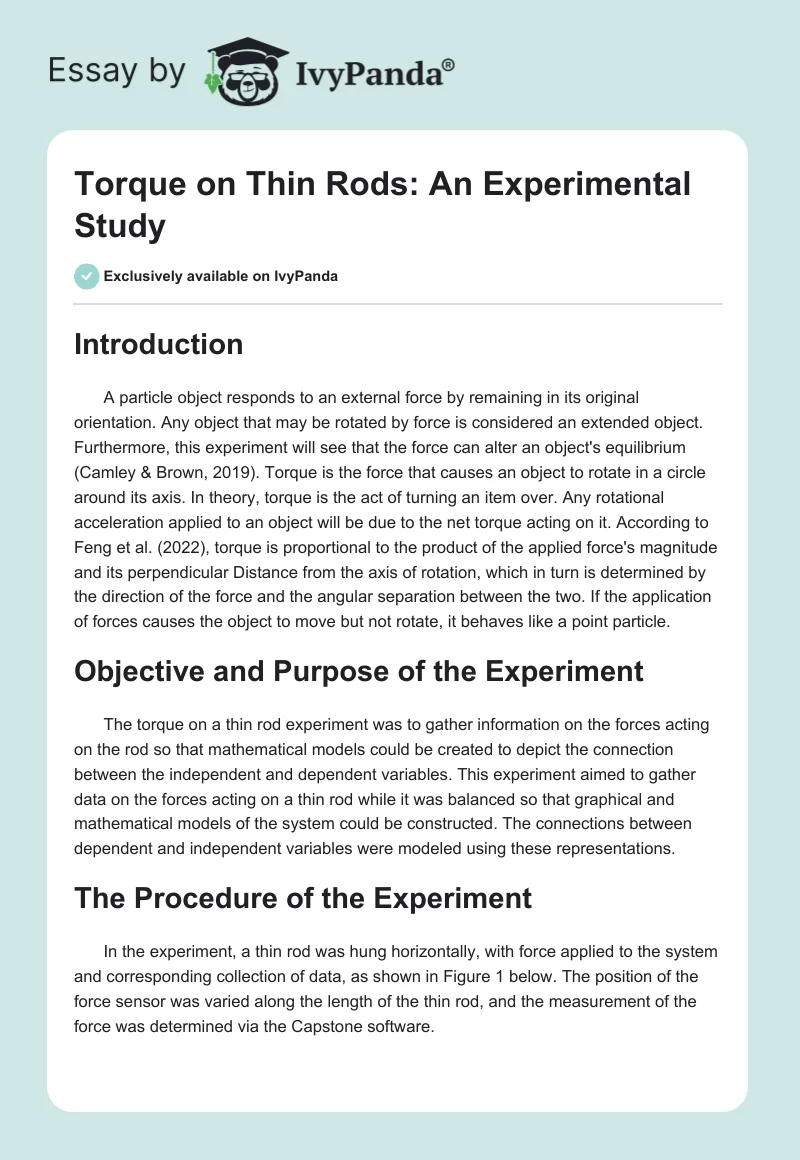
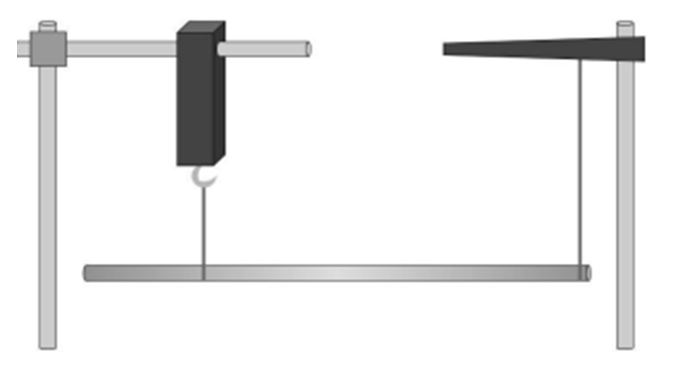
In the experiment, a thin rod was hung horizontally, with force applied to the system and corresponding collection of data, as shown in Figure 1 below. The position of the force sensor was varied along the length of the thin rod, and the measurement of the force was determined via the Capstone software.
Data Collection
A set of ten observations of the force sensor (N) were recorded at different Distance (m) along the rod as shown in Table 1 below:
Table 1: Distance and Force
Another set of seven observations was collected and recorded, as shown in Table 2 below:
Table 2: Torque and Acceleration
Analysis
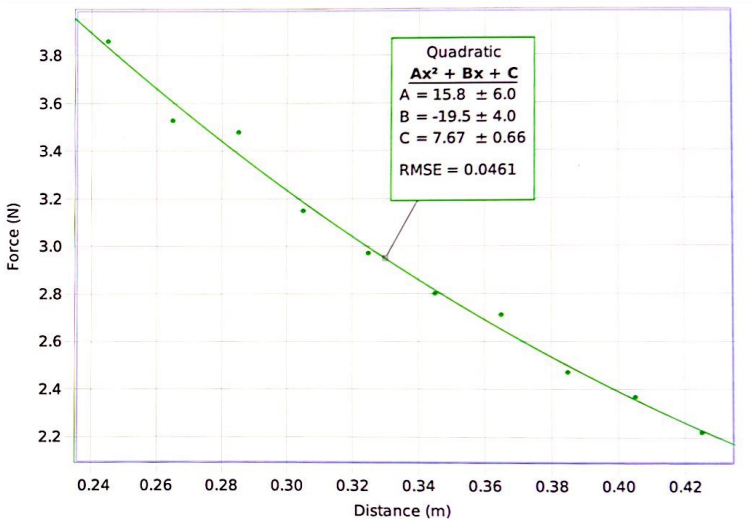
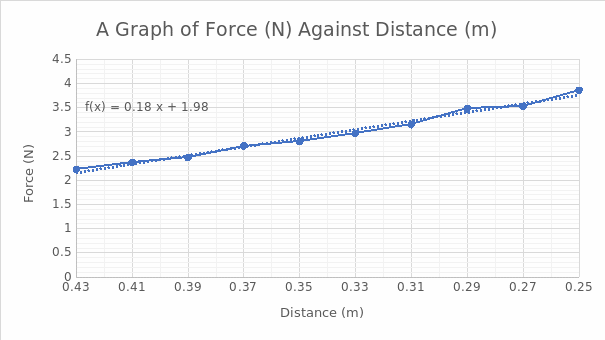
From the data collection, a graph of sensor force versus Distance is developed, leading to a polynomial equation of ![]() as shown in Figure 2 below.
as shown in Figure 2 below.
From the equation, linearized data is obtained and used to draw a linearized graph as shown in Figure 3 below.
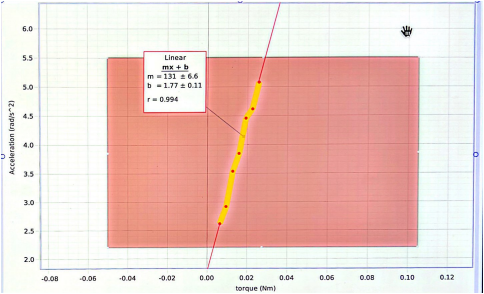
In addition, a graph of acceleration against torque is plotted as shown in Figure 4 below.
Discussion
It is discovered through this experiment how force can transform an object from a motionless to a moving one. In addition, a force does not affect the rotational motion of a point particle, but it does affect the equilibrium state of a longer item when applied. The correlation between dependent and independent variables was also studied. The vertical axis represented the independent variables, and the horizontal axis represented the dependent variables (Selvan & Balasundaram, 2021). Finally, in this experiment, various mathematical and graphical systems were used to analyze the acquired data and how familiarity with some basic forms is necessary for properly using the experiment’s data.
Conclusion
Torque was demonstrated in this experiment based on Newton’s second law. The law states that when a force is applied to an object, the object accelerates in the direction of the force. When an object is in rotational motion, however, rather than translational motion, it is set to be in rotation by the pivot point. Data obtained from torque testing on a thin rod is used to develop mathematical models. The data is represented graphically by drawing a curve from the data. A line of best fit is approximated from the data and used to obtain linearized data. In this report, the linearized data was used to provide an equation for the graph.
References
Camley, B. A., & Brown, F. L. (2019). Motion of objects embedded in lipid bilayer membranes: Advection and effective viscosity. The Journal of Chemical Physics, 151(12), 124104. Web.
Feng, G., Zhang, C., Zhang, H., & Li, W. (2022). Theoretical and Experimental Investigation of Geomagnetic Energy Effect for LEO Debris Deorbiting. Aerospace, 9(9), 511. Web.
Selvan, C., & Balasundaram, S. R. (2021). Data Analysis in Context-Based Statistical Modeling in Predictive Analytics. In Handbook of Research on Engineering, Business, and Healthcare Applications of Data Science and Analytics (pp. 96-114). IGI Global. Web.