Abstract
When two bodies collide they either stick together or separate. It is believed that there is a correlation between the initial and final momentum as well as the initial and final energy. Theoretically it is believed that in an inelastic collision both energy and momentum are conserved unlike perfectly inelastic collision where only momentum is conserved (Drexler 24). Hence, the purpose of this experiment was to ascertain this theory.
In this experiment, two carts on a runway were set to collide as the data of their velocities before and after collision were being recorded. Their velocities and masses were altered momentarily and the readings recorded. For the case of a perfectly inelastic collision, the carts were set to collide on the ends bearing Velcro. The experimental result revealed less than 20% and more than 40% lose in KE for elastic and perfectly inelastic collision respectively with some disparities in measurements. The momentum lose was kept at less than 10% for both collisions. These loses in KE could be attributed to heat, sound, deformation or light. Perfectly inelastic collision has relatively greater loses than elastic collision because it liberates more energy in many forms. Thus, the experiment almost agreed with the theoretical concept had it not been for some unavoidable uncertainties and hence, 100% momentum and/ or energy conservations are unattainable.
Experimental Objectives
The objective of this experiment is to ascertain that when bodies are involved in an elastic collision, both the energy and the momentum are conserved unlike in a perfectly inelastic collision where only the momentum is conserved.
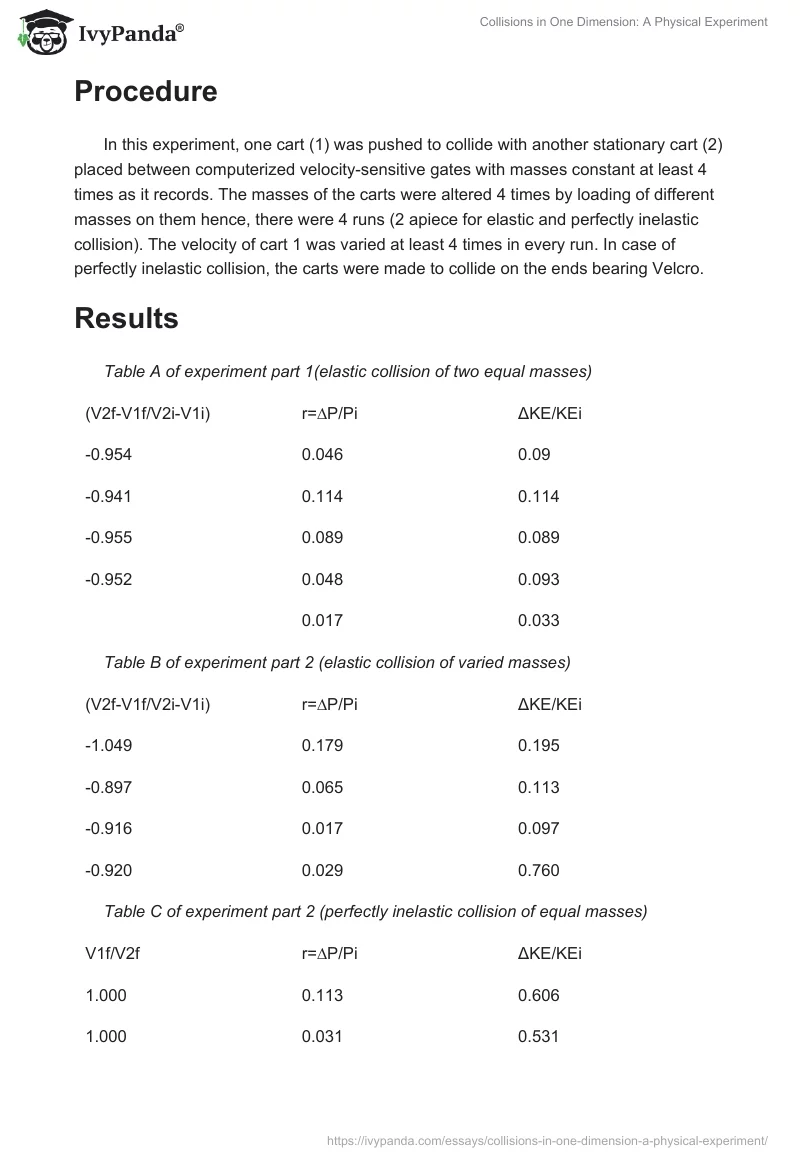
Procedure
In this experiment, one cart (1) was pushed to collide with another stationary cart (2) placed between computerized velocity-sensitive gates with masses constant at least 4 times as it records. The masses of the carts were altered 4 times by loading of different masses on them hence, there were 4 runs (2 apiece for elastic and perfectly inelastic collision). The velocity of cart 1 was varied at least 4 times in every run. In case of perfectly inelastic collision, the carts were made to collide on the ends bearing Velcro.
Results
Table A of experiment part 1(elastic collision of two equal masses)
Table B of experiment part 2 (elastic collision of varied masses)
Table C of experiment part 2 (perfectly inelastic collision of equal masses)
Table D of experiment part 2 (perfectly inelastic collision of varied masses)
Data analysis
For elastic collision, both the energy and the momentum is conserved hence,
- Therefore, m1v1i+m2v2i =m1v2f+m2v2f
- Rearranging the equation we get: m1 (v2f-v1i) =m2 (v2i-v2f)
- But, 1/2m1v1i^2+1/2m2v2i^2 =1/2m1v2f^2+1/2m2v2f^2
Rearranging and simplifying the energy equation:
- m1 (v2f^2-v1i^2) =m2 (v2i^2-v2f^2)
- m1 (v2f-v1i)(v2f+v1i) =m2 (v2i-v2f)(v2i+v2f)
Dividing momentum equation by energy equation;
- (v2f-v1f) = – (v2i-v1i)
- Dividing both sides by (v2i-V1i) we get; (v2f-v1f)/ (v2i-v1i) = -1
Table 1 of experiment part 1(elastic collision)
Relative velocities were obtained from the quotient of (V2f-V1f)/ (V2i-V1i).
Therefore, (0.374-0)/ (0-0.392) =-0.954
r=∆P/Pi= [(P1f+P2f)-(P1i+P2i)]/ (P1i+P2i)
= [(m1V1f+m2V2f)-(m1Vi+m2iV2i)]/ (miVi+m2iV2i)
= [(0.524*0+0.524*0.374)-(0.524*0.392+0.5237*0)]/ (0.524*0.392+0.524*0)
= 0.046
ΔKE/KEi = (KEf –KEi)/KEi
= [(0.5*m1*V1f^2) + (0.5*m2*V2f^2)] – [(0.5*m1*V1i^2) + (0.5*m2*V2i^2)]/ [(0.5*m1*V1i^2) + (0.5*m2*V2i^2)]
= [(0.5*0.524*0^2+0.5*0.524*0.374^2)-(0.5*0.524*0.392^2+0.5*0.524*0^2)]/ (0.5*0.524*0.392^2+0.5*0.524*0^2)
= 0.090
Table 2 of experiment part 2 (elastic collision)
Relative velocity ratio= (0.254-(-0.11))/(0-0.347)=-1.049
r=∆P/Pi = [(0.500*(-0.110)+1.022*0.254)-(0.500*0.347+1.022*0)]/ (0.500*0.347+1.022*0)
= 0.179
ΔKE/KEi = [(0.5*0.500*(-0.11)^2+0.5*1.022*0.254^2)-(0.5*0.500*0.347^2+0.5*1.022*0^2)]/ (0.5*0.500*0.347^2+0.5*1.022*0^2)
=0.195
Table 3 0f experiment part 3 (perfectly inelastic collision)
V1f/V2f= 0.197/0.197=1
r=∆P/Pi = [(m1+m2) Vf-(m1Vi+m2iV2i)]/ (miVi+m2iV2i)
= [(0.524+0.524)*0.197-(0.524*0.444+0.524*0)]/ (0.524*0.444+0.524*0)
= 0.113
ΔKE/KEi = [(0.5*(m1 + m2)*V^2)] – [(0.5*m1*V1i^2) + (0.5*m2*V2i^2)]/ [(0.5*m1*V1i^2) + (0.5*m2*V2i^2)]
= [(0.5*(0.524 + 0.524)*0.197^2)-(0.5*0.524*0.444^2+0.5*0.524*0^2)]/ (0.5*0.524*0.444^2+0.5*0.524*0^2)
= 0.606
Table 4 of experiment part 4 (perfectly inelastic collision)
V1f/V2f= 0.096/0.096=1
r=∆P/Pi = [(m1+m2) Vf-(m1Vi+m2iV2i)]/ (miVi+m2iV2i)
= [(0.500+1.022)*0.096-(0.500*0.325+ 1.022*0)]/ (0.500*0.325+ 1.022*0)
= 0.104
ΔKE/KEi = [(0.5*(m1 + m2)*V^2)] – [(0.5*m1*V1i^2) + (0.5*m2*V2i^2)]/ [(0.5*m1*V1i^2) + (0.5*m2*V2i^2)]
= [(0.5*(0.500 + 1.022)*0.096^2)-(0.5*0.500*0.325^2+0.5*1.022*0^2)]/ (0.5*0.500*0.325^2+0.5*1.022*0^2)
= 0.735
For perfectly inelastic collision in part 4, rexpected= (m-M)/2m
= (0.500-1.022)/2*0.500
= -0.522
Discussion
The objective of this experiment was to affirm that in deed when bodies involve in an elastic collision, both the energy and the momentum are conserved. However, this is not the case with perfectly inelastic collision where only the momentum is conserved. From the experiment; with respect to elastic collision as shown in table 1 and 2, the relative velocity ratios were approximately equal to the expected (-1) with extremes being -0.897 and -1.049. For perfectly inelastic collisions, the ratio V1f/V2f was constant since the bodies are moving with a common velocity.
For the elastic collision (part and 2), the ∆P/Pi remained fairly below 10% with only two values exceeding 10%. This was expected however, this disagrees with the theory which states that the momentum ought to be conserved 100% and hence ∆P/Pi should be equal to zero (Drexler 23). The fluctuation in momentum can be attributed to lose of energy due to sound or friction on impact. Similarly, for perfectly inelastic collision the momentum loses were fairly below 10% with an extreme value of 53%.
As regards energy conservation, elastic collision recorded energy loses below 20% with the only extreme value hitting a high of 75%. The 20% energy loss is expected since on impact some energy is lost as sound, and the friction encountered as a result of moving parts as well as the surface runway. Comparatively, perfectly inelastic collision recorded greater losses than the former. The energy losses were greater than 40%. This is so because more energy losses are involved e.g. deformation, heat, light or sound.
The uncertainties that occurred while taking the experiment have a bearing on the equipments used. Some of the errors could be due to the friction on the moving parts and the surface. However, this can be minimized in future by using frictionless pulleys and surfaces. For easy adjustments to minimize the influence by gravity the run-way ought to be placed on sliding wedges. Moreover, the deviations in the data are partly contributed by the design of the experiment which didn’t put enough precautions like: the conditions under which the experiment ought to be done. It is imperative to carry out the experiment in an area where the wind flow is calm since this can hinder the smooth flow of the carts and thereby alter the results.
Conclusion
As attested by the data and the analysis done here in, the experiment gave satisfactory results that reflect the objective of the experiment. This is so because we did ascertain that indeed the energy and the momentum for both types of collision almost agreed with the theoretical values had it not been for experimental uncertainties.
Works cited
Drexler, Jerome. How Dark Matter Created Dark Energy and the Sun: An Astrophysics
Detective Story. Makawao, Maui, HI: Inner Ocean Publishing, 2003. Print.