A probability distribution lists all possible values for a random variable alongside their probabilities. If a random variable can take on specific predetermined values, then the probability distribution is discrete. Otherwise, the probability distribution is continuous. For example, the probability of getting a head or a tail when one flips a coin can be either one or zero.
This means that it is impossible to get values with decimals in Discrete Probability Distributions. For instance, it is impossible to get 2.7 heads when one a coin. On the other hand, it is possible to get values with decimals in continuous distributions.
A clearly and carefully constructed probability distribution is useful in determining many variables that may be of interest to a forecaster. For example, a car dealer may be interested in finding the probable number of cars that may visit the dealership on particular days of the week. This may be important for appropriate staffing, getting additional parking and savings costs.
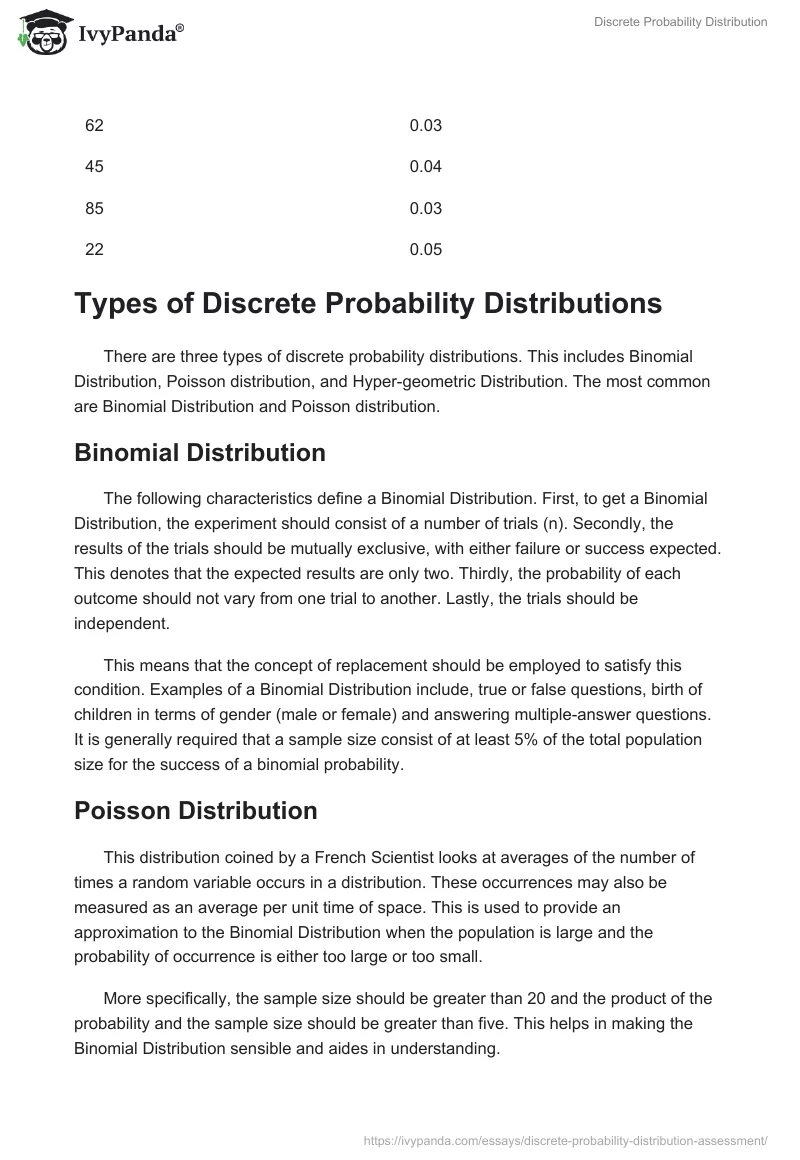
Hence, the dealership looks at the historical number of cars that visit the workshop for a month and the subsequent probabilities that the cycle will repeat itself. Discrete probability is also used in football matches where every decision that involves either of the two teams is decided using a flip of a coin. Additionally, discrete probability plays a major role in a game of cards. This is a perfect example of a discrete probability distribution where the variables are fixed. The following is an example of a probability distribution.
Types of Discrete Probability Distributions
There are three types of discrete probability distributions. This includes Binomial Distribution, Poisson distribution, and Hyper-geometric Distribution. The most common are Binomial Distribution and Poisson distribution.
Binomial Distribution
The following characteristics define a Binomial Distribution. First, to get a Binomial Distribution, the experiment should consist of a number of trials (n). Secondly, the results of the trials should be mutually exclusive, with either failure or success expected. This denotes that the expected results are only two. Thirdly, the probability of each outcome should not vary from one trial to another. Lastly, the trials should be independent.
This means that the concept of replacement should be employed to satisfy this condition. Examples of a Binomial Distribution include, true or false questions, birth of children in terms of gender (male or female) and answering multiple-answer questions. It is generally required that a sample size consist of at least 5% of the total population size for the success of a binomial probability.
Poisson Distribution
This distribution coined by a French Scientist looks at averages of the number of times a random variable occurs in a distribution. These occurrences may also be measured as an average per unit time of space. This is used to provide an approximation to the Binomial Distribution when the population is large and the probability of occurrence is either too large or too small.
More specifically, the sample size should be greater than 20 and the product of the probability and the sample size should be greater than five. This helps in making the Binomial Distribution sensible and aides in understanding.
Hyper-geometric Distribution
In Binomial Probability Distribution, the assumption is that the population size is quite large. For this reason, the probability after every trial is expected to be relatively stable. The Hyper-geometric Distribution is used in two situations. First, it is used to find the probability of a particular number of results (i.e. success or failure) when a sample is drawn from a predetermined population without replacement.
Secondly, it is used to find the probability of a particular number of results (i.e. success or failure) when the sample size exceeds 5% of the population size (i.e. n>5%*N). A predetermined population is a fixed number of objects or measurements specifically for a particular experiment. It is also referred to as a finite population.
Conditions to Satisfy Discrete Probability Distributions
A Discrete Probability Distribution, in mathematical terms, should fulfill the following conditions.
- The probability of X taking a specific value is p(X). This shows that a discrete probability function can assume an unlimited number of values.
- The second condition requires that all probabilities of X have non-negative values. This is normally true in a situation where a set of non-negative integers are involved. This is also true in situations where there are subsets. Mathematically, there is no restriction regarding the fact that all discrete functions should be integers. However, it is illogical to conclude the latter practically. For example, when one flips a coin the expected result is either a head or a tail.
- The total of all probabilities should be one. That is, after all the events have been considered the sum of probability of occurrence should be one. Each event has a probability of occurrence that ranges from 0-1. However, in any distribution, this should add up to one. This means that in any distribution, there cannot be a situation where the event does not occur. For example, when you toss a coin the expectation is that either a head or tail will show. There is no chance that none will occur. Another example is a final football match where there must be a winner one way or the other. Additionally, a gambling game represents a discrete probability situation where someone will have to win/lose.
Comparison: Discrete and Continuous Distributions
A continuous variable automatically assumes a Continuous Probability Distribution. The following are ways in which a Discrete Probability Distribution differs from a Continuous Distribution.
- The probability that a continuous variable will take on a certain explicit value is zero.
- A Continuous Probability Distribution cannot be expressed in a table. This is because of the characteristic above.
- However, a Continuous Probability Distribution uses a formula to express the different probabilities.
Discrete Probability Distributions sometimes assume the name Probability Mass Functions. On the other hand, Continuous Probability Functions are referred to as Probability Density Functions. A Probability Density Function has the following properties that distinguish it from the latter. First, random variables in a continuous function occur in a continuous range of values. This range is called the domain that defines the variables.
Hence, the density function graph will have a continuous characteristic over the range. The area under a curve of a density function is normally equal to one. This is true when calculated over a domain of the random variable. The probability that a variable will assume a value between x and y will be equal to the density function area under the curve that the two random variables form.