Before voltage can cause a current flow through resistances to perform work, a complete path or circuit is needed. During the process, electric current flows in electrical conductors such as wires, circuit board traces as well as components (Halliday and Resnick 651). What flows is the electric charge, q, which is measured in coulombs (C). The rate of charge flow is referred to as the Current, denoted as I, and is given as follows;
There are four electrical quantities namely; the charge, voltage, current, and resistance (McDermott 11). Current can be defined as the change in charge that flows past a given point per change in time over which the change in charge is measured. Current is measured in units called amperes (A) of which one ampere is one coulomb per second. The amount of current that flows when a voltage is placed is referred to as resistance.
Voltage has units of volts, V, and is an “across” quantity, such as pressure and speed. Across quantities are usually measured between two points, either junctions or nodes. Basic components of electric circuits There are several types of circuits and all require the same basic components, like power, conductors, working devices, control devices, and protection devices. A power source (battery or alternator) produces a voltage or electrical potential.
Conductors include wires and printed circuit boards and they provide a path for current flow. The working devices (also known as loads) such as lamps and motors tend to transform the electrical energy into another form of energy that can perform work. Switches and relays are control devices that are responsible for turning the current flow on and off. Finally, examples of protection devices include fuses and circuit breakers and their work is to interrupt the current path if there is an overload of current flow. However, too much current flow can damage conductors and working devices hence the use of the protection devices. There are five basic things to look for in any complete circuit. These are; the source of voltage, protection device, load, control, and ground.
Types of electric circuit Three types of circuits have been distinguished as series, parallel and series-parallel. These types of circuit are differentiated by the source of power, conductors, loads, and control or protective devices connected (Feynman, Leighton and Sands 28). Series circuit This is the simplest type of circuit. The conductors, control and protection devices, loads, and power source are connected with only one path for current while the resistance of each of these devices can be different.
In addition, the amount of current flowing through each of the devices is the same. However, the voltage across each of the devices may be different as well. If the path is broken, no current can flow (McDermott 48). Parallel circuit This has more than one path for current flow. The same amount of voltage is usually applied across all paths. If the load resistance in each path is the same, the current in each path will be the same. However, if the load resistance in each path is not the same, the current in each path will be different as well. Unlike the series circuit, if one path is broken, the current will continue to flow to the other paths. Series-Parallel circuit This is a hybrid of both series and parallel circuits. The power source and control or protection devices are usually arranged in series form whereas the loads are in parallel.
The same current flows in the series portion whereas different currents flow in the parallel portion. In addition, the same voltage is usually applied to all devices arranged in parallel portions compared to different voltages applied to all devices which are arranged in series portions. In the event, if the series portion is broken, the current stops flowing in the entire circuit. On the same note, if the parallel portion is broken, the current will continue to flow in the series portion and the remaining branches (Feynman, Leighton, and Sands 29). Use of Ohm’s law in electric circuits Ohm’s law is the most basic and often used electrical equation presented as follows; v=R*I
Resistance is defined by Ohm’s law as vI. Applying Ohm’s law to a series circuit is easy since one is required to add up the load resistances and divide the total resistances into the available voltage in order to get the current. To get the voltage drop across the load resistance, you need to multiply the current by each load resistance. Voltage drop is the difference in voltage on one side of the load compared to the voltage on the other side of the load. The voltage drop is proportional to the amount of resistance, therefore, the higher the resistance, the higher the voltage drop, and vice versa. Applying Ohm’s law to the parallel circuit is a bit difficult compared to series circuits.
This is because the path resistances must be combined to find equivalent resistance. It is worthwhile to note that, the total resistance in a parallel circuit is less than the smallest load resistance. This makes sense because the current may flow through more than one path. In addition, the voltage drop across each path will be equal since the source voltage is applied to each path. In series-parallel circuits, applying Ohm’s law is a matter of simply combining the rules for both series and parallel circuits.
First, calculate the equivalent resistance of the parallel load and add it to the resistance load of the series. The total resistance is then divided into the source voltage to find the current. To get voltage drop across the series load, you simply multiply current by resistance. In addition, the current is obtained by dividing voltage by resistance (Feynman, Leighton, and Sands 33).
Troubleshooting Accurate and fast electrical troubleshooting is quite easy when you know how voltage, current, and resistance are related. The relationship is clearly explained by Ohm’s law: I=E/R, where I is the current (amps), E is voltage (volts) and R is the resistance (ohms). Ohm’s law is very helpful when diagnosing electrical problems. For instance, when the resistance remains constant, the current goes up as well as the voltage and when the current goes down the voltage goes down. On the other hand, when the voltage remains constant, the current goes up when resistance goes down and when the current goes down the resistance goes up.
However, bypassed devices greatly reduce resistance causing high current. Loose connections increase resistance thereby causing low current. Kirchhoff’s voltage and current laws In addition to Ohm’s law, Kirchhoff,s two laws are the most basic circuit principles. Kirchhoff’s Voltage Law (KVL) states that the sum of the voltages around a closed loop must be equal to zero as defined in the equation below:
Current flows from the positive terminal of the source by convention causing voltage drops across resistors and other non-power generating circuit elements (McDermott 48). This will cause the voltage to rise since current goes into the negative terminal and out the positive terminal that is from – to + (Halliday and Resnick 653). However, voltages traversed in this direction will be negative. Dissimilarly, current flows into the positive voltage end of resistances as shown below:
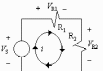
Applying KVL, ![]()
Kirchhoff’s Current Law (KCL) states that the sum of current into a terminal must be equal to zero:
In other words, charge does not accumulate in nodes. Currents flows into a node, by convention are negative and out of a node are positive. Conclusion It can be concluded that, the effect of individual resistors on the total resistance of a circuit depends on whether the circuit is series or parallel.
Work cited
McDermott, Lillian, Physics by Inquiry. Toronto: John Wiley, 1996.
Halliday, D. and Resnick, R. Fundamentals of Physics. New York: Wiley, 1988.
Feynman, R., Leighton, R. and M. Sands, Feynman Lectures on Physics. Addison–Wesley: Reading, MA, 1964.