Background
In reservoir simulation, the goal is to infer an actual reservoir’s behavior from its physical or mathematical model’s performance. While the physical model is to the scale of the original reservoir’s dimensions, a mathematical model is different. It is a set of differential equations with the proper arrangement of boundary conditions that appropriately describe the essential physical processes occurring in the modeled system (Peaceman, 1977). The mathematical model allows one to learn the fluid flow equation without having to develop a laboratory model of the entire system.
Fluid flow and mass transfer are the main processes occurring in petroleum reservoirs. On the one hand, there are up to three immiscible phases in terms of fluid flow, comprising simultaneous water, oil, and gas movement (Peaceman, 1977). On the other hand, mass transfer often happens primarily between the oil and gas phases (Peaceman, 1977). Other factors playing a role in the fluid flow process include gravitational pull, capillary rise, and viscosity.
Mathematical equations account for these forces and consider the physical system’s arbitrary description regarding its geometry and heterogeneity. Thus, one obtains the differential equation by combining Darcy’s law with a straightforward differential material balance for each phase (Heinemann, 2013). One can get solutions by the mathematical physics’ classical methods if the case is simple, namely, it involves regularly-shaped boundaries, such as a circular one about a single well. A numerical model, being in its essence a computer program, is highly effective for obtaining solutions for complex reservoir situations.
Darcy’s Law
According to Darcy’s law, the volumetric flow rate (Q) through a horizontal system’s porous material sample of L length and A cross-sectional area is given by:
Here, K is the medium’s absolute permeability, 
the fluid’s viscosity, and 
the applied pressure drop across the sample. The differential form of Darcy’s law for a flow parallel to the x axis is given by a superficial flow velocity of the form:
Here, 
is x-direction’s pressure gradient while the negative sign denotes a declining pressure in the flow’s direction. However, this equation does not consider gravitational pull. Assuming the depth D to be an arbitrary function of v’s coordinates (x, y, or z), differential Darcy’s law accounting for gravity will contain new factors p, representing the fluid’s density and g, representing the acceleration due to gravity, becoming:
Mass Conservation
The mathematical model of the simultaneous fluid flow in a porous medium often considers two immiscible fluids (oil and water) where no mass transfer exists between them. In this case, water is the wetting phase because it wets the porous medium more than oil, which is now the non-wetting phase (Chen, 2007). The wetting and non-wetting fluids are denoted by the subscripts w and o respectively. Here, mass conservation is obtained by understanding the fact that two fluids jointly fill the voids of the porous medium, that is, Sw + So = 1, where Sw + So represent the wetting and non-wetting phase saturation, respectively.
Notably, the pressure in the non-wetting phase is greater than the wetting one’s because of the two phases’ curvature and surface tensions. The two phases’ pressure difference is given by Pc = Po – Pw, where Po and Pw, are the non-wetting and wetting phases’ respective pressures (Chen, 2007). Mass accumulation per unit time in a differential volume will be given by:
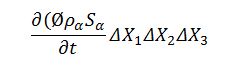
Here, the porous medium’s porosity is given by 
and the density of each phase is given by 
The cube’s length in the xi –direction is given by 
Taking this equation, if there is no mass transfer between the two immiscible phases, mass is conserved in each fluid (Chen, 2007). Still, α is either the wetting or the non-wetting phase. The equation for mass conservation is:
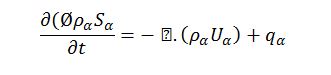
Reference List
Chen, Z. (2007). ‘Chapter 5: Two-phase flow and numerical solution’, in Chen, Z. (ed.). Reservoir Simulation Mathematical Techniques in Oil Recovery. Philadelphia: Society for Industrial and Applied Mathematics, pp. 83-99.
Heinemann, Z. (2013) Systematic of reservoir flow equation. Leoben: Association for Promotion of Scientific Work in Reservoir Characterization and Simulation.
Peaceman, D. W. (1977). ‘Chapter 1: Differential equations for flow in reservoirs’, in Peaceman. D. W. (ed.). Fundamentals of Numerical Reservoir Simulation. New York: Elsevier Scientific Publishing Company, pp. 1-34.