Introduction
Different research articles have pointed out that the mean heights of the people aged 20 years and above are 69.3 and 63.8 inches for both the male and female population respectively. However, this research was conducted to verify the results based on a hypothesized mean of 66.5 inches to either accept or reject the null and alternative hypotheses. The data that was used in the study was collected from a sample of the target population using questionnaires and analyzed using the one-sample t-statistic test.
Assignment task
Identify a research problem
The goal of the research problem was to determine whether the mean height of adults aged 20 and above was 63.8 inches for the females and 69.3 inches for the males as noted in various scientific articles such as the report contained in the CDC Growth Charts (Washington, Karlaftis & Mannering, 2010). Here, tests were conducted on the data to determine the veracity of the claim. The null and alternative hypotheses were stated as follows:
The hypotheses
- H0 (Null hypothesis) = 66.5: (The mean height is equal to 66.5)
- H1 (Alternative hypothesis) ≠ 66.5: (The mean height is not equal to 66.5)
Research plan
The data that was used in the study was collected from the target population to create a sample size that was analyzed and the results generalized across the population of interest. Male and female participants aged 20 and above constituted the target population and the sample.
A simple random sampling technique was applied to identify the sample size from the target population. The sample size was calculated by using statistical software for determining the sample size from a large population of people aged 20 and above. A margin of error of approximately less than 2.5% was thought to be appropriate for this study. As is the case in most research surveys, the study used a 95% confidence interval to show that the findings did not happen by chance but were logically deduced and reliable (King, Rosopa, & Minium 2010). A test statistic was done using SPSS to determine the z-score (Z score = 1.96) based on the margin of error (confidence interval) already mentioned. However, 0.5 was used as the most forgiving number and it was taken as the standard deviation in the study.
Analyzing data
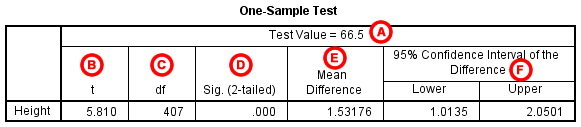
The data was collected, properly coded and entered into the SPSS software for analysis. However, it was necessary to avoid type I and type II errors that usually occur due to errors in interpreting the null and alternative hypotheses respectively (Pratt & Cullen, 2000). It was common to assume that the population had a normal distribution, which made it possible to use the central limit theorem to interpret the results of the test-statistic on the sample used in the investigation. A hypothesized mean value was entered into the application window as a test value and compared with the mean of the data sets to determine the t-statistic. The mean value, t-statistic, the degree of freedom, and confidence interval were determined to complete the statistics as shown in table 1.
Table 1
Tools for data collection
The tool that was used for data collection is a closed-ended questionnaire because the variable, height, is continuous and the persons selected for the study were required to select one value as their height. A quantitative research paradigm was the foundation of the research because numerical data was used for statistical analysis.
Justification
The one-sample t-test is appropriate for comparing the mean between two values, one being a hypothesized mean and the other the statistical mean and that could be appropriate to accept or reject the null and alternative hypotheses. The closed-ended questionnaire could enable the respondents to select values that the researcher will find easy to analyze.
Literature review
The research problem was modeled on both the null and alternative hypotheses using height as a continuous variable (Rodgers, 2010). King et al. (2010) argue that by using a one-sample t-test, it is possible to determine the difference between the mean of the two values and use them to either reject or accept the null and alternative hypotheses on 95% confidence interval because the probability of a correct answer is 0.95 (Coladarci, Cobb, Minium & Clarke, 2010). The data used in the study is characterized as being continuous, independent, lacking outliers, and normally distributed. However, in most instances, t-statistic can be used to analyze data that is not normally distributed. It is possible to apply a lower and upper bound of the confidence interval on the dataset to ensure the reliability of the findings.
Enders (2010) notes that the independent sample t-test is appropriate for comparing two mean values of the heights of people aged 20 and above without reference to specific people.
Conclusion
It is evident from the study that the null and alternative hypotheses could be accepted or rejected based on the t-statistic test. The statistical analysis was done to determine the difference between the population mean and the hypothesized mean using a one-sample test. By interpreting the results, the hypotheses could be proved correct or wrong.
References
Coladarci, T., Cobb, C. D., Minium, E. W., & Clarke, R. C. (2010). Fundamentals of statistical reasoning in education. New York: John Wiley & Sons.
Enders, C. K. (2010). Applied missing data analysis. Guilford Press.
King, B. M., Rosopa, P. J., & Minium, E. W. (2010). Statistical reasoning in the behavioral sciences. New York: Wiley Global Education.
Pratt, T. C., & Cullen, F. T. (2000). The empirical status of Gottfredson and Hirschi’s general theory of crime: A meta‐analysis. Criminology, 38(3), 931-964.
Rodgers, J. L. (2010). The epistemology of mathematical and statistical modeling: a quiet methodological revolution. American Psychologist, 65(1), 1.
Washington, S. P., Karlaftis, M. G., & Mannering, F. L. (2010). Statistical and econometric methods for transportation data analysis. New York: CRC press.