Introduction
According to Heiman, a z-score denotes a number of standard deviations from the mean, which can be either positive, if the raw score is above the mean, or negative if the initial data index is below the mean (Heiman, 2003, p. 94). Arising from this, the presented assignment provides several variables.
First, 17 minutes is an average time that Eric takes to get to work. 3 minutes is a standard deviation that is needed to drive from home, park the car and to get to his job. 21 minutes is time that Eric once needed for getting to this work. Therefore, it is considered as a raw score. According to formula z-score equals to:
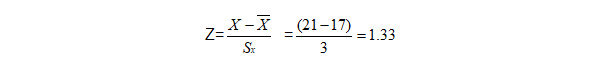
The calculations have revealed that z-score is positive because the initial time taken by Eric is above the established mean.
Calculations
If the time Eric gets to his work equals to 12, the calculation of z-score will look as follows:
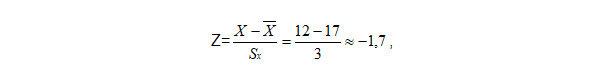
where X is the raw score, is the mean, and Sx equals to standard deviations. The calculations have revealed that the z-score is negative because the initial data is below the established mean.
Finally, if the raw score is equal to 17, it is possible to expect that the z-score will be identical with the raw score. Arising from the above data, z-score will be equal to zero (0) because it is similar to raw value. This can be illustrated by the formula:
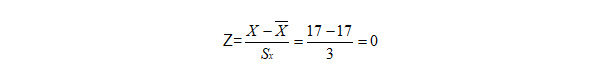
By converting the data into z-value, it is possible to define the relative probabilities of various scores for average quantities. It also allows to estimate the variations of two different points that dependent on the same mean. Finally, z-scores reveal lower and higher distributions within a datum unit.
Discussion
The above-presented calculations provide variations in standard deviation from the average distribution score. Due to fact that z-score identifies a raw scores’ location taking into consideration the extent to which it is above or below the mean as presented in standard deviations, the calculated z-scores (1.33, -1.7, and 0) allows use to define to what extent each variable correlates with the same raw score. Although they represent dimensionless quantity, they are still based on number derived from individual raw data (Howitt and Crammer, 2007 p. 46).
It should be admitted that positive and negative values of Eric getting to work identifies the speed with which he can traverse the distance from home to work in accordance with the established normal standard deviation. It provides a possibility to evaluate and calculate the time needed for passing the distance and establishing the norms.
Due to the fact that normal distribution curve show a number of data distributions with regard to the average mean, z-scores identifies the deviation from the norms and evaluates the extent the data deviations from the normal distribution.
In this regard, a normal curve is a set of values for the mean. Despite this fact, normal curves are based on the same property because standard deviation establishes a constant proportion for the allocation of scores.
Consequently, if it is known that a number of scores has a normal distribution and that the standard deviation and the mean are also identified, it is possible to define how many scores can be presented within particular limits. Arising from this, both concepts are closely interdependent.
Reference List
Heiman, G. (2003). Essential Statistics for the Behavioral Sciences. US: Wadsworth Publishing.
Howitt, D., and Crammer, D. (2007). Introduction to Statistics in Psychology. UK: Pearson Education.