Abstract
Atomic spectra occur when atoms and ions undergo excitation. The excitation causes electrons to vibrate in their energy levels, and thus them to move from one quantum level to another. During excitation, electrons emit different forms of light, which vary in terms of wavelength. Spectroscope can separate stream of light into different colors using diffraction grating. Thus, analysis of the diffracted light shows that colors that exists in a stream of light vary according to their wavelengths.
Introduction
The formation of the atomic spectra occurs when atoms or ions intermittently absorb and emit energy due to the excitation of the electrons. For example, when atoms or ions gain energy in the form of heat, electrons in them changes their quantum levels from ground state to an excited state. Since the change of quantum levels is intermittent, there is an emission of light in form of spectral lines. To measure atomic spectra, spectroscope is necessary. Spectroscope measures atomic spectra by generating a stream of light using a slit (entrance slit).
After generating stream of light using entrance slit, spectroscope then allows the stream of light to pass through diffraction grating (lens or prism). The diffraction grating then diffracts the stream of light and separates it into atomic spectra according to their respective wavelengths. If the angle of diffraction (θ) and the distance between lines on a diffraction grating (d) are known, then one can calculate the wavelength using the equation (λ = dsinθ). To determine unknown wavelengths using standard wavelengths, calibration plot is necessary.
Procedure
The experiment used spectroscope to determine wavelengths of the visible colors. The spectroscope comprised of the cigar box, which has a slot, a source of light, a diffraction grating, and a graph-paper scale. According to Grossie and Underwood, the slot is an entrance slit that allows 1mm of light beam to pass into the box and hits diffraction grating at an incidence angle of 30° (287). Since the beam of light diffracts as it leaves diffraction grating, an observer can view the diffracted light on a graph-paper scale, and note the readings.
In the experiment, when the observer viewed the diffracted light, red, yellow, green, blue-green, blue, and violet are sequential colors that are visible on the graph-paper scale. The procedure of the experiment deviated from printed procedures because the position of the graph-paper scale and observer is unique. However, the experiment did not present any new spectra lines.
Data and Results
Table 1: Calibration with Helium.
The table 1 shows relationships among color, graph-paper scale reading, and wavelength of spectral lines in nm.
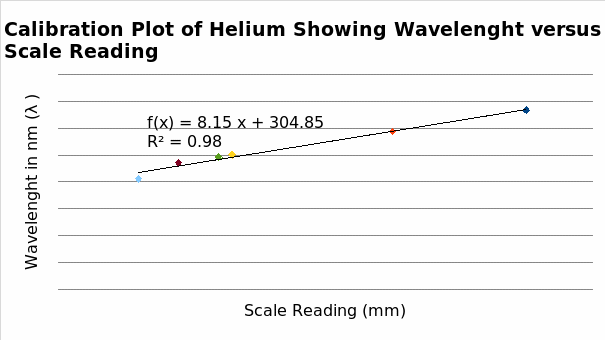
The above figure (Figure 1) is a calibration plot of Helium showing the relationships between wavelength (nm) and graph-paper reading scale (mm). By use of the calibration plot, one can establish wavelength of a given spectral line, which has specific scale reading.
Table 2: Experimentally Observed Hydrogen Spectrum Lines.
Table 3: Calculated Hydrogen Spectrum Lines.
Sample calculation of the wavelength (λ)
Given Rydberg constant as 1.0968 x 107 m-1, m = 2, and n = 3 for red color, calculation of wavelength follows the following procedure.
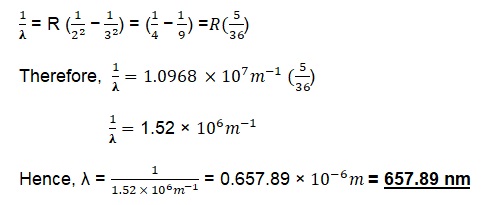
Sample calculation of the error
Error = (calculated λ – experimental λ)
Hence, error for red color = (657.89 – 675) = -17.11
Sample of the percent error
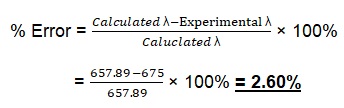
Table 4: Experimentally Observed Neon Spectrum Lines.
Table 5: Experimentally Observed Argon Spectrum Lines.
Table 6: Experimentally Observed Krypton Spectrum Lines.
Discussion
The scale reading of the spectroscope displayed wavelengths that correlated with the standard wavelengths in terms of nanometer. Derived wavelengths from the calibration plot depicted the relationship between scale reading and the wavelengths of gases such as hydrogen, neon, argon, and krypton. From the standard plot or calibration plot, red light showed experimental wavelength of 675nm in hydrogen and neon. The wavelength is within the range of 625nm to 740nm for the red light. However, neon produced orange light with an experimental wavelength of 520nm, which is not within the normal range of 590 to 625nm.
Neon, argon, and krypton produced a yellow light with wavelengths of 590nm, 490nm, and 480nm respectively. The wavelengths of the yellow light are not within the range of 575nm to 585nm that is in literature except for neon (Grossie and Underwood 286). Green, green-blue, and violet are some of the lights, which depicted their wavelengths to be consistent with literature values. Green light has a wavelength of 540nm for argon, 570nm for krypton, 560nm for hydrogen and neon. These wavelengths are closely within the range of 491nm and 575nm, a range for the green light (Grossie and Underwood 286). Moreover, blue-green and violet are other lights that depicted their wavelengths within the ranges that are available in the literature.
The uncertainties in measurements of the hydrogen atom are random because of the irregular variation in the errors. The percent error for red light is 2.60%, green light is 15.19%, blue-green is 24.4%, and violet is 2.39%. Grossie and Underwood suggest that variation in calculated and experimental wavelengths occurs because quantum energy levels vary from one color of light to another (289). Hence, the variation in wavelengths from one form of light to another is due to differences in quantum energy levels.
The differences between the experimental and literature values of wavelengths occur due cumulative effect of errors. Firstly, the design of the spectroscope is prone to errors, which affects interpretation of the readings on a given scale. Secondly, the capacity of an individual to observe colors and read the scale is another factor that contributes to errors. Thirdly, the accuracy of calibrating the spectroscope and drawing of the calibration plot also contributes to the errors, which cause experimental values of wavelengths to differ from literature values.
Conclusion
When atoms and ions gain energy, they become excited and begin to emit light. The nature of emitted light is dependent on the excitation state of the atoms and ions coupled with the nature of atom or ions. Usually, excited atoms emit red, orange, yellow, green, blue-green, blue, and violet lights with wavelengths of about 670nm, 580nm, 520nm, 500nm, 490nm, 470nm, and 410nm respectively. Hence, the use of calibration plot depicted the existence of variation between calculated wavelengths and experimental wavelengths.
Works Cited
Grossie, David, and Kirby Underwood. Laboratory Guide for Chemistry. 7th ed. New York: Hayden-McNeil, 2010. Print.