Introduction
All operations in mathematics depend on real numbers. It is, therefore, correct to assert that complex real numbers help in the derivation of complex numbers. A deep understanding of the real numbers and, indeed, complex numbers are crucial to carrying out any mathematical operations in any field.
To further understand the meanings attached to real and complex numbers, this essay will focus on brief but detailed comparisons between the two classes of numbers in mathematics. Additionally, the essay will use some graphical presentations of the theoretical explanations to enhance understanding.
Real Numbers
A real number is any quantitative representation along a continuum (Denlinger, 2010, p. 3). The best demonstration of real numbers is the one-dimensional number line. Real numbers include integers, fractions that are rational numbers but not integers, decimals, square roots, and some algebraic expressions. The figure below illustrates some of the common real numbers.
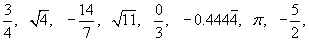
Some of the most basic properties of real numbers include rationality and irrationality and algebraic or transcendental. Rational numbers can also be positive or negative, or zero. It is important to note that real numbers mainly measure continuous quantities. One of the distinctive properties of real numbers is the real number binomials. Through the binomial theorem, it is possible to prove that power ‘r’ is a real number using expressions such as the one below.
f (x) = (1+x)r = a0 + a1x + a2x2 + a3x3 ….akxk ……
Complex Numbers
Real numbers form an important part of complex numbers. According to Kasana (2005, p.2) complex numbers are pairs of ordered real numbers. Simply put, complex numbers consist of a real number and an imaginary number that needs mathematical solving for its completion. While real numbers are straight forward, complex number systems more often than not represent an expression of the form.
For instance, a+bi represents a complex expression where ‘a’ and ‘b’ are real numbers, and ‘i’ represents an imaginary unit whose value will help in solving the complex expression. Any number whose square is a real number less than zero is an imaginary number. The square of an imaginary number is always negative. When the imaginary number, for instance, ‘bi’ is added to a real number, the result is a complex number. It is therefore correct tom assert that imaginary numbers are non-zero complex numbers with zero as the real part. The following are some examples of complex numbers.
2 + 5i, 6 +22 i, 12 – i
The ‘i’ in complex numbers are not real. The definition of the imaginary unit is as follows:
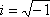
Then,
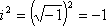
One of the most distinct differences between real numbers systems and complex number systems is the complexity of a complex number as perpetuated by its parts.
One of the most commonly used expressions in mathematics is a+bi=z. This expression makes the typical complex number. The real number part of the above expression is ‘a’, which mathematicians refer to as the real part of ‘z’. The other real number ‘b’ is the imaginary part of the complex number.
Despite their differences however, both real numbers and complex numbers take intractably interact in mathematical operations. While operations involving real numbers seem simple, operations involving complex numbers are quite involving. Operations involving both addition and subtraction of real and imaginary parts of the summand numbers make the basic procedure. The following expression illustrates the addition definition of operations involving complex numbers.
(a+bi) + (c+di) = (a+c) + (b=d)i
A graphical representation of for instance, the sum of 3 + i and –1 + 2i whose answer is 2 + 3i will be as follows;

The formula for multiplication of complex numbers mainly makes use of the square of the imaginary unit. The following expression shows the definition for the multiplication involving complex numbers (Kasana, 2005, p.2).
(a+bi)(c+di) = (ac-bd) + (bc+ad)i. For example,
The result of the multiplication between (3 + 2i) and (4 + 5i) will be as follows:
(3 + 2i)(4 + 5i) = (3 × 4) + (3 × (5i)) + ((2i) × 4) + ((2i) × (5i))
= 12 + 15i + 8i + 10i ²
= 12 + 23i -10 (Note that 10i ² = 10(-1) = -10)
= 2 + 23i
Therefore, (3 + 2i)(4 + 5i) = 2+23i.
The following a graphical illustration of complex number multiplication of the equation (1+i)*(-1+i)=-2
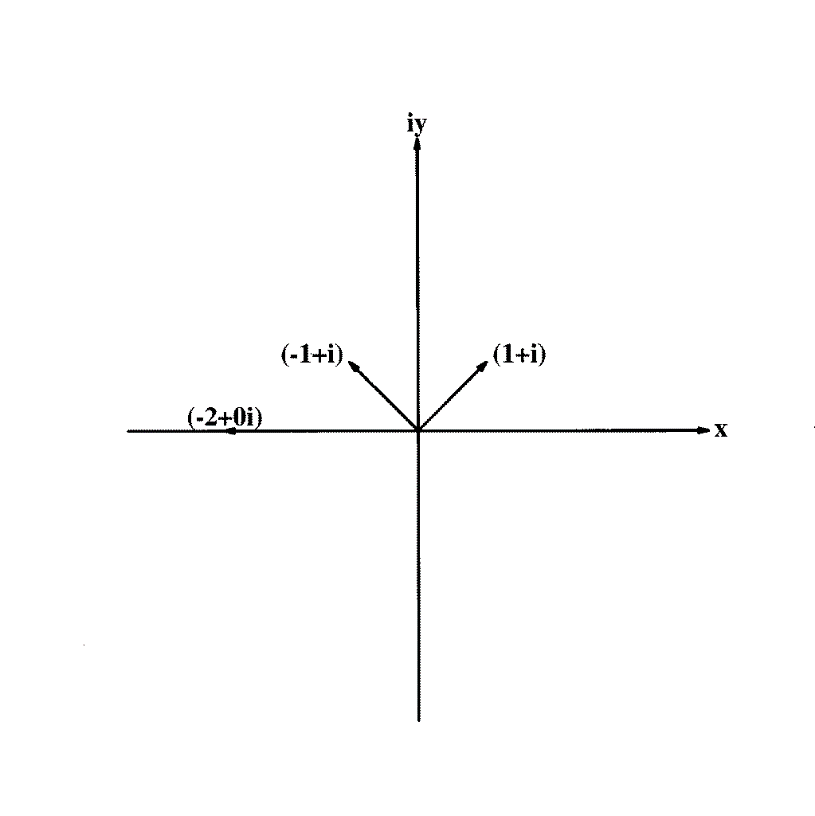
One of the most dominant contrasts between real and complex numbers is their use and representation on the number line. Unlike real numbers, complex number systems enable the extension of the one dimension number line. While real numbers uses the conventional number line, complex numbers use the two-dimensional complex plane that the Argand diagram illustrates.
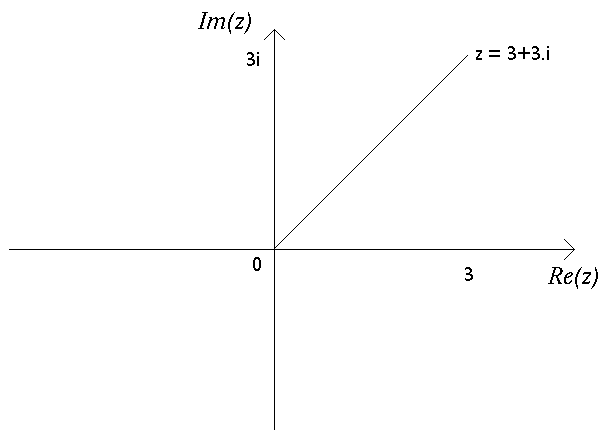
In a nutshell, any analysis of the two systems of numbers will yield many more differences and similarities whose origin is the operations involving the numbers in the two systems. This therefore was a theoretical approach that only gives a face value comparison.
References
Denlinger, C. (2010). Elements of Real Analysis. New York: Jones & Bartlett Learning.
Kasana, H.S. (2005). Complex Variables: Theory And Applications 2Nd Ed. London: PHI Learning Pvt.